| 00:00 | Ah where, where were we, left off yesterday in the middle of |
|
| 00:07 | uh the final chapter, final lecture classical ra waves and rays, which |
|
| 00:15 | a lot of complications. And, know, there's lots more complications we |
|
| 00:20 | go on with this for a long time. Uh But we're gonna |
|
| 00:25 | uh stop. Uh we only have little bit more in that lecture to |
|
| 00:28 | . And uh um uh also we your questions. So let's uh let's |
|
| 00:37 | up your questions and uh uh address first. No. OK. So |
|
| 00:50 | first one is from Carlos, it , how does the funnel zone influence |
|
| 00:57 | design considerations for 3D seismic acquisition? huh. Uh uh That's a very |
|
| 01:06 | question. And so uh uh are you involved in um 3d acquisition |
|
| 01:13 | ? Yeah, partially I have been . Yeah, a couple of |
|
| 01:18 | Yeah. Uh uh So uh it an evolving topic and uh the main |
|
| 01:25 | to say is that uh the uh the effort we put out on the |
|
| 01:30 | is increasing every year dramatically. Uh that we acquire lots more traces. |
|
| 01:37 | Let me just tell you a story um uh from my uh teenage |
|
| 01:45 | I was a teenager growing up in Texas. And, you know, |
|
| 01:49 | Texas is one of the great oil of the world comparable to the giant |
|
| 01:55 | in uh Saudi Arabia. And my before me was a geophysicist and he |
|
| 02:02 | uh he was what we call a bugger. Uh uh That was uh |
|
| 02:06 | a name they gave to a geophysicist maybe in the 19 thirties, 90 |
|
| 02:12 | ago. And uh uh so, those days, um uh we were |
|
| 02:19 | learning how to do exploration geophysics by and error in the field. And |
|
| 02:25 | course, uh those were uh days two D acquisition and of course, |
|
| 02:31 | could not have dreamed of the kind uh issues that you uh just |
|
| 02:37 | Carlos. Let me tell you one uh when I was a teenager, |
|
| 02:41 | came home from the office very And I said, what uh uh |
|
| 02:48 | what happened at the office? And said, well, the crew managed |
|
| 02:53 | acquire 100% data, 100% coverage that in and, and uh uh uh |
|
| 03:03 | in, in modern language, we call that single fold data, single |
|
| 03:08 | coverage. Imagine that he was so when he finally had uh enough data |
|
| 03:14 | uh to have a single fold. this was actually before, before uh |
|
| 03:21 | midpoint uh gathering was invented. So had just, um, a few |
|
| 03:27 | , maybe 20 receivers, something like . And he, uh, only |
|
| 03:32 | few sources and he had to spread the sources. Uh, so to |
|
| 03:37 | uh as to cover the, the , of course, it was two |
|
| 03:40 | line. Uh, and he had spread the further apart than the, |
|
| 03:45 | , uh fren zone, uh, would, uh, uh, |
|
| 03:51 | And when he finally got, uh, enough equipment in the field |
|
| 03:56 | sort of in the middle of his , finally got enough uh uh equipment |
|
| 04:02 | he could put on the ground or could achieve uh uh a single fold |
|
| 04:11 | with uh uh the F Fornell Zone included. Isn't that remarkable? And |
|
| 04:17 | today, we might have 1000 so my father could not have conceded |
|
| 04:24 | . Now, the Fornell Zone can very large, very uh uh uh |
|
| 04:32 | um if you look at the formula uh included in there is the uh |
|
| 04:38 | depth to the reflector. So at, at large depth, say |
|
| 04:42 | ft, the reel zone can be large. Uh So today, uh |
|
| 04:51 | we uh commonly almost always have so sources and so many receivers on the |
|
| 04:59 | at one time that we uh uh don't really uh design. Uh We |
|
| 05:06 | really consider the fernell zone limitations in design. We consider other things, |
|
| 05:12 | example, uh uh we'll, we'll um uh the fold which we |
|
| 05:19 | uh, and we'll consider the, , the range of Ainu, |
|
| 05:25 | when we have wide auth acquisition. , then, uh, how should |
|
| 05:30 | arrange that, uh, in, a marine environment? There are, |
|
| 05:34 | , limited options. Um, if doing tow streamers, uh, you |
|
| 05:40 | have a second shooting boat off to side or you might have, |
|
| 05:45 | well, you can think of lots different, uh, considerations, you |
|
| 05:49 | . Uh, an amazing one is uh called a Coral shooting. Uh |
|
| 05:56 | , do you, uh know about shooting, uh offered by Washington? |
|
| 06:02 | . Yes. II, I actually on some of those. That's, |
|
| 06:08 | mean, interpreting because I, I interpretation, but I, I was |
|
| 06:13 | on some of those. So just fill in everybody, uh, and |
|
| 06:17 | shooting was invented by a smart guy the Western Chico. I, you |
|
| 06:21 | , I know him but I forgot name. Do you, do you |
|
| 06:23 | the name Rosada? No, I , I don't very guy and he |
|
| 06:28 | this about 10 years ago. And the ship does not sail in a |
|
| 06:33 | line. It sails uh uh in . It's sort of like a, |
|
| 06:37 | moving Helix. And so behind the, the, the streamer is |
|
| 06:42 | kilometers long and it's sort of trailing a Helix. And as he, |
|
| 06:47 | doesn't go round and round in a , he, he, he moves |
|
| 06:51 | as he makes his circles. So uh like a Helix. And that |
|
| 06:56 | out to provide uh uh a good asthma coverage with a, with asimos |
|
| 07:05 | distributed that is, uh, uh most, uh, uh, let |
|
| 07:12 | back up. Uh, if you a, a wide asthma uh code |
|
| 07:17 | , uh with another shooting boat off the side, then you'll always find |
|
| 07:22 | , uh, that there are certain which are uh not present in the |
|
| 07:28 | , you look at a common midpoint of that kind of white asthma data |
|
| 07:32 | you'll find that it sure enough, has uh many asthmas ever uh in |
|
| 07:38 | data set, but there will be angles where there just aren't any and |
|
| 07:44 | uh uh those uh angles will, be different or maybe as a function |
|
| 07:49 | offset. So uh uh in Qu shooting, uh you don't have that |
|
| 07:55 | have uh asthma in all directions and of them are both short and long |
|
| 08:02 | in all directions. But it makes a complicated geometry to figure all that |
|
| 08:08 | and to present to do the And uh I think it makes it |
|
| 08:14 | uh well, there are are certain in and interpretation, but I'm not |
|
| 08:22 | get into that here. But uh guess the, the, the point |
|
| 08:26 | that in 3d acquisition um design, in wide and as there are so |
|
| 08:32 | um so many considerations to uh the, the gist had to think |
|
| 08:40 | . Um and he uh uh normally not concern himself with Fornell zone |
|
| 08:46 | And the reason is because he has many receivers on the ground at, |
|
| 08:51 | one time. So he automatically uh all the uh uh uh it would |
|
| 09:00 | made fell very happy and we are see shortly and within the next |
|
| 09:05 | we're gonna see uh shortly how um um OK, the modern techniques overcome |
|
| 09:19 | uh limitations that uh were perceived to a serious limitations in my father's |
|
| 09:28 | So, uh uh you, you can anticipate uh uh further refinements |
|
| 09:36 | technique. Uh For, for here's another refinement technique which is just |
|
| 09:43 | uh uh realized is uh simultaneous So if you have uh uh say |
|
| 09:48 | source boats, uh we used to that you had to uh time the |
|
| 09:54 | so that uh one shot doesn't interfere the other shot or suppose there was |
|
| 10:01 | company uh doing their own survey a miles away. You had to come |
|
| 10:06 | an agreement with that company that uh gonna shoot between two and three o'clock |
|
| 10:10 | you can shoot between three and four , that sort of stuff, time |
|
| 10:15 | with the shooting. And uh that an expensive business when, when |
|
| 10:21 | um delay shooting for any reason. It seemed like only a few seconds |
|
| 10:29 | or a few minutes, but it up and uh, it can |
|
| 10:34 | um, uh, the cost of survey increase substantially. Professor. |
|
| 10:40 | uh, regarding that, uh, apart should the votes or if, |
|
| 10:45 | you said, two different companies are for different areas, how apart they |
|
| 10:52 | be? So they don't interfere because some point it would interfere, |
|
| 10:57 | Even if they are very, very apart. So how, what would |
|
| 11:00 | like a decent distance such a We can, uh, we don't |
|
| 11:06 | to do that today. We know to affect how to correct for those |
|
| 11:12 | and process because we can see that wave vectors from that other quote that |
|
| 11:17 | want to ignore, those are coming from another direction and we know they're |
|
| 11:21 | coming in from our source. So have techniques for uh you know, |
|
| 11:26 | uh FK filtering and things like that um uh uh to eliminate those other |
|
| 11:34 | from our data. But it wasn't so obvious how to do that. |
|
| 11:38 | I remember in, in, in day, I was shooting some ocean |
|
| 11:42 | seismic surveys in the early days of bottom seismic. This would have been |
|
| 11:47 | the late 19 nineties. So maybe the time you were being born, |
|
| 11:52 | was sitting on a boat in the of the North Sea uh acquiring ocean |
|
| 11:58 | seismic data. And about 20 miles , there was another crew uh working |
|
| 12:03 | a different company and they were doing same thing or maybe they were doing |
|
| 12:07 | seismic anyway. We did not know those days how to, uh, |
|
| 12:12 | those shots from our data. So , uh, uh, made an |
|
| 12:18 | with that other company. Uh, shoot between two and three o'clock and |
|
| 12:22 | shoot between three and four o'clock. in the meantime, we just sat |
|
| 12:26 | , uh, uh, uh, the water just bobbing up and down |
|
| 12:29 | for them to be shooting and the is ticking and everybody is getting paid |
|
| 12:34 | , uh, uh, the capital of the equipment is, uh, |
|
| 12:39 | ha uh, having to be It was an expensive business. And |
|
| 12:44 | , uh, uh, uh, think that people in western Chico were |
|
| 12:49 | responsible for realizing that we could eliminate shots coming in from, uh, |
|
| 12:56 | from the other direction in processing is don't rely on the other, on |
|
| 13:02 | distance. We don't, uh, , uh, um, uh, |
|
| 13:08 | , uh, the, the, , the shots are easily, |
|
| 13:12 | uh, detectable 2030 miles away. , uh So it's not the distance |
|
| 13:19 | we utilize to, uh, uh, get rid of that, |
|
| 13:27 | , uh, other manmade noise. do it, uh, because of |
|
| 13:32 | , uh, of the direction the are coming in. And, |
|
| 13:37 | so we can do, uh, can apply the same thing on |
|
| 13:41 | We can have uh uh multiple vibrator operating uh uh in the same patch |
|
| 13:48 | desert in Abu Dhabi, for And uh, so they acquire thousands |
|
| 13:54 | thousands, uh, of, shot points every day because they can |
|
| 13:59 | , um, um, that they have, uh, multiple vibrator crews |
|
| 14:09 | , you know, within a mile each other. But, uh, |
|
| 14:12 | , uh, uh, the, waves and that other, uh, |
|
| 14:16 | are, uh, and, and shooting at the same time, |
|
| 14:20 | you know, wi within a second two of, of our shooting. |
|
| 14:24 | , but we can eliminate those arrivals because we know which direction they're coming |
|
| 14:29 | and we can filter out those uh in, in that way, very |
|
| 14:34 | and very uh uh uh in a uh uh advance in the economics of |
|
| 14:42 | of acquisition. This is called uh simultaneous shooting. And it, |
|
| 14:48 | it, it, it's usually it's done simultaneously, but it can be |
|
| 14:53 | within a second or two of, each other. And still we uh |
|
| 14:57 | get rid of those uh sounds that don't want uh because we know where |
|
| 15:02 | coming from. So uh those are considerations that we uh uh consider uh |
|
| 15:10 | acquisition design. Um And not so the Fornell Zone considerations because those are |
|
| 15:19 | care of automatically because we have so receivers on the ground. Oh, |
|
| 15:27 | . Uh The there is another uh issue here which I'll mention in, |
|
| 15:34 | connection with converted waves in converted Uh You know, the image point |
|
| 15:41 | not at the midpoint. The image is uh closer to the receiver |
|
| 15:46 | And furthermore, the image point uh from the source, it varies with |
|
| 15:54 | . We talked about this uh Whereas where uh deeper reflections uh deeper |
|
| 16:02 | uh reflective conversions happen closer to the than shallow. So what this means |
|
| 16:11 | that too uh design a, a converter wave survey, you basically, |
|
| 16:17 | can't do it in your head, have to have software to do it |
|
| 16:22 | the software has to be uh properly uh designed. And I think most |
|
| 16:28 | them are designed properly these days, the effects of anisotropy. So it |
|
| 16:34 | out that the uh that anisotropy does a big effect on where this converted |
|
| 16:41 | converts. I think we'll be talking that next Friday. OK. But |
|
| 16:49 | uh that's the long answer to Carlo's is uh uh normally we have a |
|
| 16:54 | more things on our mind than the . Thank you, Professor. Let's |
|
| 17:01 | what else we have? Oh, , here's one from Shelley. Uh |
|
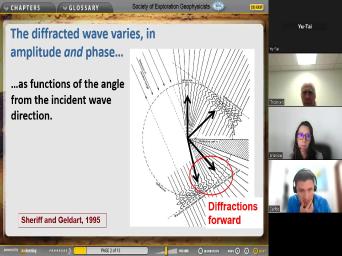
| 17:06 | question is on slide 51. As quotes here. The diffracted wave varies |
|
| 17:13 | amplitude phase assumptions of the angle uh the infinite wave. And so |
|
| 17:20 | she goes on, that's a quote the slide on 51. And uh |
|
| 17:25 | says, my question is which direction the largest amplitude. So let us |
|
| 17:30 | pull up that very uh uh that slide. Hold on a second |
|
| 17:37 | Um I, I can do this quite quickly. I hope. Um |
|
| 17:48 | here I am in that oh, and uh OK. So, um |
|
| 18:04 | I'm gonna put this into presentation mode then I am going to minimize that |
|
| 18:14 | share with Dal Matt Sly. Um . So I think everybody sees |
|
| 18:34 | the slide by showing the diffraction. . And so uh uh uh I |
|
| 18:40 | the answer to your question, Lili right here on the slide. See |
|
| 18:44 | diffraction here. Uh oh Excuse let me get a pointer. Here's |
|
| 18:50 | partner. OK. See these diff here behind the, the point. |
|
| 18:56 | here are the, the um uh the arrivals uh of uh uh which |
|
| 19:03 | the uh miss the de factor And over here are the uh are |
|
| 19:09 | reflected uh waves. And uh uh think uh you have identified um uh |
|
| 19:19 | mistake in the uh in the figure to my eye, these reflections have |
|
| 19:27 | same amplitude as the unreflective instant wave . So, of course, there's |
|
| 19:34 | be a reduction in a, in um because of the reflection coefficient. |
|
| 19:41 | So, uh th this is a by uh Sheriff and Gil dot |
|
| 19:47 | I'm, I'm sorry to see you know, the Sheriff uh was |
|
| 19:51 | prominent member of the faculty in this , University of Houston for many, |
|
| 19:56 | years. And the book is a pretty good book, but this |
|
| 20:03 | , it's a mistake for us to these reflection amplitudes with these transmitted amplitudes |
|
| 20:09 | uh are not interacting with the, the D fracture at all. But |
|
| 20:16 | , let's consider uh uh uh uh range of events. Uh I think |
|
| 20:20 | legitimate to look at this range of . And we can see that uh |
|
| 20:25 | diffracted arrivals here uh uh They're not back at the, at this uh |
|
| 20:32 | the reflection angle is this one. these here are pure diffraction and here |
|
| 20:38 | begin to interfere with the uh And here is a pure reflection way |
|
| 20:44 | here. So this sequence of, uh uh waveforms uh uh as accurate |
|
| 20:52 | relative amplitudes. So you can see the, the fractions are, are |
|
| 20:57 | smaller. And furthermore, there are uh uh waveform. This waveform here |
|
| 21:03 | different from that. So uh the to your question is the fractions are |
|
| 21:09 | . Um No. Um uh we on a subsequent slide, I'm gonna |
|
| 21:30 | forward a couple of slides here. . Uh uh Again, you can |
|
| 21:37 | the diffraction are less here. These are less here than the pure |
|
| 21:48 | But remember Hagen's principle says that you construct a reflected wavefront out of |
|
| 21:57 | many diffraction along this line. So you have a diffraction uh uh right |
|
| 22:02 | , putting out um a spherical uh like this and then another one close |
|
| 22:08 | another one close by and so on of those uh add up just like |
|
| 22:11 | see here. I just drew four them here because there's a million of |
|
| 22:15 | . Now, you can see they add up to make a reflective |
|
| 22:19 | So um uh the uh uh let's talk about the amplitude of these |
|
| 22:29 | along this curve here. Um I the amplitudes, if you look at |
|
| 22:35 | waveforms, these are just the arrival here. Uh uh If you just |
|
| 22:39 | , look at the waveforms, you'll that the one which is here's |
|
| 22:43 | the, the practice choice point for circular wave. And so this is |
|
| 22:50 | uh angle of reflection here, that will be a strong diffraction and this |
|
| 22:57 | will be a weaker diffraction and so . And uh so those all um |
|
| 23:02 | up uh to make a, a wavefront as an equivalent way of thinking |
|
| 23:11 | uh uh reflections. I actually prefer this way of thinking of uh of |
|
| 23:20 | Hagen's way of thinking, but I the way we did it before. |
|
| 23:25 | . So let me uh minimize this , and you can probably see it |
|
| 23:32 | my screen. It's minimized. I now looking back at, at the |
|
| 23:40 | my inbox. And so we have a question from Mesa, she says |
|
| 23:47 | slide 61 why diffracted arrival with a of LAMDA over two would have opposite |
|
| 23:58 | . OK. So let us go that slide right here. Um Thank |
|
| 24:08 | , I'm I'm still sharing the Um Is that correct? Yeah. |
|
| 24:20 | . So uh I wanna stop right . So uh uh uh Carlos can |
|
| 24:25 | see right in here that the formula the radius of the reel zone as |
|
| 24:30 | there, the depth as well as wavelength? So when the depth is |
|
| 24:36 | , this term dominates and it can very locked. Uh uh But uh |
|
| 24:43 | we design a survey, we usually concern ourselves too much with the um |
|
| 24:51 | size of that Fornell zone radius. gonna go forward. OK. This |
|
| 24:56 | what uh mead is asking about. this one here has a one way |
|
| 25:01 | delay of 1/8 of the um uh of the wavelength I shouldn't call it |
|
| 25:11 | delay as it has an extra path , more than this, the extra |
|
| 25:15 | length is wavelength divided by eight. two times that is a wavelength divided |
|
| 25:20 | four. That is the uh what uh fernel uh defined to be the |
|
| 25:29 | of the first fernel zone. And course, you can define uh larger |
|
| 25:34 | zones. Uh uh uh But that's one which we normally, when we |
|
| 25:39 | the Fornell Zone, usually we mean first Fornell Zone. Now in red |
|
| 25:45 | , it says if the defra arrival a delay of delta over two, |
|
| 25:50 | one has the delay of delta over . I'm saying it wrong. This |
|
| 25:53 | not a delta, this is a per case lambda, this is the |
|
| 25:59 | so that if it has half uh half a wavelength, uh it would |
|
| 26:04 | uh opposite polarity. Well, you that uh when you have AAA sine |
|
| 26:09 | , uh it goes from a peak trough to a peak, that's one |
|
| 26:13 | and half a wavelength is a peak trough. So if this, if |
|
| 26:18 | ray ray is coming in with an extra path length of, of |
|
| 26:22 | half a wavelength, it's gonna be a trough where this one has a |
|
| 26:27 | so that it's going to uh add , they're gonna superpower line um uh |
|
| 26:35 | we discussed before because the wave equation a linear equation, they're gonna spose |
|
| 26:42 | just add them up and that uh from this uh arrival uh coming with |
|
| 26:49 | extra path length of half or wavelength gonna uh uh be arriving at the |
|
| 26:54 | time as the uh as the peak the direct ar. So I, |
|
| 27:02 | I understood correctly, so the other add uh constructively because the delay is |
|
| 27:09 | minor. So they add to each and this is the opposite because it's |
|
| 27:14 | of the OK. OK. And also put in the right weasel |
|
| 27:19 | it's, it's partial uh in construction the waves in here and partial destruction |
|
| 27:26 | the waves coming from out here. . So, uh of course, |
|
| 27:32 | it's a smooth transition, right? It gets to be uh um uh |
|
| 27:38 | it's, it's a wa waves reflecting my ear are very constructive. |
|
| 27:47 | they uh have uh mostly the same the, the, the peak is |
|
| 27:52 | in almost the same time as this here. And that um uh |
|
| 27:56 | the inaccuracy of the superposition increases steadily you go all the way out here |
|
| 28:03 | fell, just picked this arbitrary point to be uh um So it makes |
|
| 28:11 | a two way additional way path of uh landed over four while showing |
|
| 28:18 | lamb, I sh showing the one uh extra length. Uh But it's |
|
| 28:23 | to do two ways extra length and just picked that number, uh glammed |
|
| 28:30 | four as a compromise between zero and over two here. Ok. So |
|
| 28:41 | , uh let us then just um pick up where we left off. |
|
| 28:50 | I, I'm going to, um think the easiest thing to do is |
|
| 28:54 | to step through this. Oh, , we didn't have far to |
|
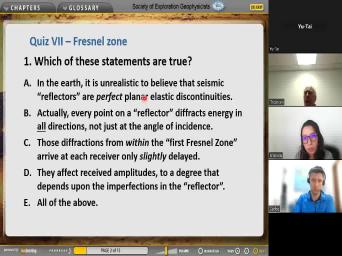
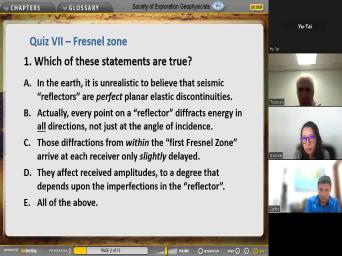
| 28:58 | Ok. So, uh we, left off with this um uh quiz |
|
| 29:04 | and so, um let's uh let just show you, uh we're, |
|
| 29:10 | about to change topics right here, I left it uh at this |
|
| 29:15 | So let me turn to Carlos uh say which of these statements are true |
|
| 29:22 | notice here at the bottom we got of the above. So, um |
|
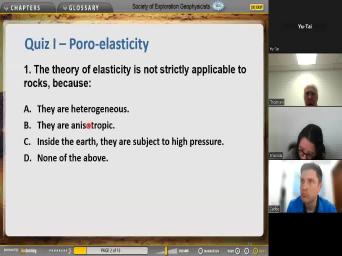
| 29:26 | A is it in the earth? it unrealistic to believe that seismic reflectors |
|
| 29:32 | perfect plain planar elastic cont notice. you say that's true or false? |
|
| 29:37 | would say it's true, professor. , of course, of course. |
|
| 29:42 | uh here's a a um uh you know, as geophysicists, we |
|
| 29:48 | make approximations and we always uh make approximations. And so all of our |
|
| 29:55 | about a vo assumes that they are those reflectors are perfect planar reflector. |
|
| 30:03 | you just said they're not, and course, they're not, they, |
|
| 30:06 | , they are the results of a process, uh uh uh a sedimentary |
|
| 30:12 | . And uh uh it's bound to an imperfect mirror. So we should |
|
| 30:17 | about that whenever we're doing a VL uh at the, at the very |
|
| 30:23 | of the A VL analysis, we an assumption which is obviously not |
|
| 30:30 | we assume that reflector is a perfect reflector. And we also of |
|
| 30:36 | assumed that the incoming wave was a plan or wave and we know that |
|
| 30:40 | of them are not true. So need to approach any a vo uh |
|
| 30:47 | with a certain humility uh and looking the data and, and I, |
|
| 30:52 | showed you um um a workflow that had in BP which uh managed to |
|
| 31:00 | find the uh the anomalous fluids in subsurface. Despite uh uh the shortcomings |
|
| 31:09 | the analysis, the workflow was robust those shortcomings. And maybe this shortcoming |
|
| 31:20 | . But it is uh it, , it is important to uh uh |
|
| 31:23 | that in mind. And I uh of the reasons I'm, I'm gonna |
|
| 31:31 | back here, let me go Uh And yeah, this right |
|
| 31:35 | So if uh we talked about this , this is a converted wave, |
|
| 31:40 | common midpoint converted wave gather with one multiplied by minus one. So to |
|
| 31:45 | it symmetrical, but it, and is more or less symmetrical, but |
|
| 31:50 | got copious energy arriving here at a incident, which according to our theory |
|
| 31:57 | not happen. So when we have a disconnection between the data and the |
|
| 32:04 | data always wins, right? The is oversimplified. Now, maybe the |
|
| 32:10 | so uh to analyze this kind of , you cannot analyze this kind of |
|
| 32:15 | using a standard um uh theory for reflections because of the copious energy reflected |
|
| 32:28 | at uh uh zero offset. So have to analyze this sort of |
|
| 32:37 | Uh You need to generalize a theory some way which I nobody really |
|
| 32:43 | I have a couple of ideas. uh uh who knows um uh what's |
|
| 32:50 | . The important thing here here is the particular data set here, but |
|
| 32:54 | the realization that whatever is causing this converted energy is taking that energy away |
|
| 33:03 | the other outgoing waves. And it be a miracle if none of the |
|
| 33:09 | energy coming in here, none of was robbed from the reflected P |
|
| 33:14 | which is the one that we're most in. So uh when we solve |
|
| 33:19 | problem, then immediately we should turn attention to say, OK, what |
|
| 33:24 | that do to the uh reflected B uh is that going to affect our |
|
| 33:32 | analysis in an important way or And uh so it could be very |
|
| 33:39 | . Uh I should say that this of data is not everywhere. |
|
| 33:44 | most converted wave data uh more or obeys the simplified theory, but this |
|
| 33:51 | doesn't. And uh uh here it that over here data like this is |
|
| 33:55 | , I would say data like we find in uh say 10 or |
|
| 33:59 | of the cases. So if you , so that means that probably means |
|
| 34:05 | for those same 10 or 20% showing C wave data, they also show |
|
| 34:14 | P wave data. And what should , should we be worried or |
|
| 34:18 | I think we should be worried. think this is uh uh uh going |
|
| 34:23 | be a um uh I think we solve this sort of problem. If |
|
| 34:29 | solve this sort of problem, we uh uh learn a great new insight |
|
| 34:35 | regard to P wave A BL. so this is not the sort of |
|
| 34:40 | that can be uh taken up by student at a university or a professor |
|
| 34:50 | a university because they probably don't have data in their hand to show |
|
| 34:55 | Instead, this has gotta be taken by, by people like Bea and |
|
| 35:00 | colleagues at Schlumberger. Uh uh Somebody gonna uh be looking at this kind |
|
| 35:05 | data inside, you know, acquired western Chico. And uh uh they're |
|
| 35:10 | say to their boss that, you , we ought to figure this |
|
| 35:14 | Why don't you send me to the of Houston to work with the professors |
|
| 35:19 | and we'll figure it out. And , uh uh Flu Rose is a |
|
| 35:23 | good company, pretty good at supporting kind of um, oh, |
|
| 35:30 | proper proposals from their own people. so maybe they'll say sure, go |
|
| 35:36 | it, go for it. We'll you uh uh two years to, |
|
| 35:39 | do that. Uh You're gonna keep , your job here at plumbers and |
|
| 35:43 | the same time, you're gonna have full time job at Western Chico and |
|
| 35:47 | have had a full time job, full time study at your age. |
|
| 35:52 | um uh uh but you're young, can do it and at the, |
|
| 35:58 | we'll furthermore, we, we'll pay the uh tuition at your age. |
|
| 36:03 | also we'll uh uh uh let you our data on a proprietary basis and |
|
| 36:08 | two years you'll come back and you'll us, OK, I figured out |
|
| 36:12 | it is and uh uh uh how affects our P wave analysis for our |
|
| 36:18 | other customers for P, for our bo customers. And, uh you'll |
|
| 36:25 | glad that you spent the money that on me. That's gonna happen either |
|
| 36:30 | Schlumberger or some other uh service company has a lot of data like this |
|
| 36:35 | maybe even an o an old company has data like this. Uh And |
|
| 36:40 | worried about that for their particular uh maybe, uh uh they, maybe |
|
| 36:47 | have, uh maybe they can see the A vo the P wave Avio |
|
| 36:56 | that they have is anomalous in some . And they wonder could it be |
|
| 37:01 | of this or something else? uh uh could be uh this or |
|
| 37:07 | be something else. And my, favorite explanation for anomalous A bo behavior |
|
| 37:15 | an iso me which we'll take up week. So let us proceed here |
|
| 37:24 | issues of resolution. So, um course, we are limited in our |
|
| 37:34 | in lots of ways and everybody all the interpreters say to the processors |
|
| 37:43 | uh uh give me more resolution. you know, if, when we |
|
| 37:49 | them more resolution, uh it's uh more confusing, you know, because |
|
| 37:55 | subsurface doesn't look like these cartoons that uh draw for ourselves. The subsurface |
|
| 38:01 | is complicated and so maybe you want uh look past those complications or maybe |
|
| 38:09 | want to look more closely at Uh It's uh it's not obvious that |
|
| 38:15 | always want more and more resolution because more resolution we have, the more |
|
| 38:23 | we see. So uh and then uh the the more doubts we |
|
| 38:30 | the more doubts we have about whether not the that complexity is real. |
|
| 38:35 | let me uh uh begin this discussion uh we can, we can only |
|
| 38:41 | about these issues of resolution deeply if look at a particular data set, |
|
| 38:48 | I don't wanna do, I just give you general considerations here. |
|
| 38:56 | So most of this discussion has been about plane waves, you know, |
|
| 39:03 | waves go on forever. Um but our, our data uh has |
|
| 39:12 | uh wavelets. No, it doesn't plane waves in it. But what |
|
| 39:17 | did was we uh analyze the reflection these uh uh by the way, |
|
| 39:24 | have only a single frequency in them they go on forever. Um Using |
|
| 39:31 | as an input, we uh uh analyzed the reflection and the transmission found |
|
| 39:38 | what the coefficient should be. And uh uh luckily we found that these |
|
| 39:47 | were independent of frequency. And what means is you can uh uh take |
|
| 39:53 | incoming um uh plane waves, them the reflection and the outgoing uh uh |
|
| 40:01 | is gonna be a plane wave, you can uh add those up uh |
|
| 40:06 | both the incident wave and the function , add them up uh using a |
|
| 40:11 | synthesis and it's gonna have the same here we go, the wavelet is |
|
| 40:20 | of many such waves which combine to a waveform, which is local, |
|
| 40:24 | is localized in time. And because these coefficients are independent of frequency, |
|
| 40:31 | uh the wavelet has the same way as incoming. And uh and why |
|
| 40:38 | that because all the frequencies reflect equally that scenario of an isolated reflector. |
|
| 40:52 | , if there's a another reflector nearby uh as well as it, there's |
|
| 40:57 | thin bed and, and uh we looking at the, the top Ooo |
|
| 41:02 | that thin bed. Well, at bottom, there's gonna be uh uh |
|
| 41:06 | bed, another reflection, those uh reflections from nearby interfaces are gonna superpose |
|
| 41:16 | the reflections uh that we just uh were discussing from the top of the |
|
| 41:22 | . And so uh that's gonna make , a combined reflection which is more |
|
| 41:31 | than the, the uh simple analysis indicate. Yeah, what determines the |
|
| 41:39 | of this wavelength. Well, uh it's determined by the source itself, |
|
| 41:45 | know, whether it's a dynamite or air gun or Viber size. This |
|
| 41:49 | is particularly interesting. Both of these impulsive. This one is not |
|
| 41:56 | The uh you know, the Viber vi vibrates for a period of several |
|
| 42:00 | . So it's putting out uh uh uh vibrations that last for several |
|
| 42:07 | But we have clever techniques which uh Joe will tell you about to convert |
|
| 42:12 | this into effectively an impulsive source just that. So normally we don't have |
|
| 42:21 | single source point, we have multiple source points. For example, |
|
| 42:25 | on a V size crew, there be five trucks and they drive along |
|
| 42:31 | line and they get to the shot , they call it a shot |
|
| 42:34 | Uh, although there's no shooting uh, but that comes from |
|
| 42:39 | from the dynamite days. That was , in my father's day. And |
|
| 42:43 | when I, when I first uh my first job in this |
|
| 42:47 | I worked on a field crew and were using dynamite. Sure enough. |
|
| 42:51 | the dynamite would go off. Uh the um shot hole, the dynamite |
|
| 42:56 | be about 50 ft down and the hole would be filled with water and |
|
| 43:02 | the shooter would push the button and dynamite would go off and the uh |
|
| 43:07 | would squirt out of the hole up a height of about like 100 |
|
| 43:11 | So, uh uh we were all to stay away from the shot hole |
|
| 43:14 | that happened. So, uh that's the bad old days. Uh And |
|
| 43:18 | we don't have dynamites. We still it a shot point. So imagine |
|
| 43:23 | have a driver size field crew with trucks and they, uh and so |
|
| 43:29 | , they drive up to the shot . Uh so that the, the |
|
| 43:33 | truck in line stops exactly on the where the shot point is the others |
|
| 43:39 | nose tail right close to the, , uh, to that number three |
|
| 43:45 | or maybe spaced further apart. The can decide whether he wants the source |
|
| 43:51 | as close as possible or maybe, , uh, uh, spread further |
|
| 43:57 | . That's a design consideration for land . Also, he might not want |
|
| 44:04 | five in the line. He might , uh, uh, the, |
|
| 44:08 | , one directly over the shot 0.1 front, one in back and one |
|
| 44:12 | each side. So uh that's easy do in some circumstances and hard to |
|
| 44:18 | in other circumstances. But you can that uh whoever is designing the survey |
|
| 44:24 | want to do that. So all those are, are the source array |
|
| 44:30 | . Uh Then after, after that fires off down goes the downgoing |
|
| 44:37 | But as it's going down, uh propagates uh uh loses energy and loses |
|
| 44:43 | frequency by attenuation as the topic of next uh lecture. And also by |
|
| 44:49 | the uh the fact of friendly multiples uh the down going primary with a |
|
| 44:58 | delay. And so that changes the of the, of the wavel. |
|
| 45:01 | talked about that uh yesterday and it's uh affected by uh uh reflections from |
|
| 45:08 | interfaces. That's the issue of um resolution which we take up right |
|
| 45:17 | So how are we gonna define Well, we can define it uh |
|
| 45:21 | terms of space or time and uh it's the minimum separation between two |
|
| 45:27 | which permits confident determination that they really two features, not one. So |
|
| 45:34 | see that's sort of a fuzzy you'll see what we mean by |
|
| 45:38 | Uh um But uh it has to fuzzy because um of the this effect |
|
| 45:47 | , of uh of uh near of nearby features, our analysis of that |
|
| 45:54 | depends upon the means we use to and also to analyze the data. |
|
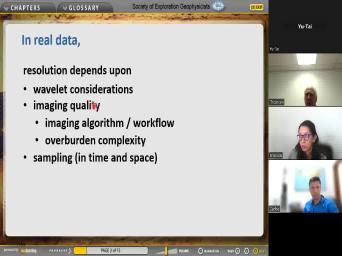
| 45:59 | in real time, um the resolution real data, the resolution depends upon |
|
| 46:05 | sorts of wavelength considerations and also the uh workflow. So we're not gonna |
|
| 46:14 | looking at a single reflection um to single receiver. No, we're going |
|
| 46:19 | be putting together uh events from uh uh different sources and receivers and uh |
|
| 46:31 | a um a a people grave we're, we're gonna arrange for them |
|
| 46:34 | to have a common image point. this collect uh within the gather of |
|
| 46:40 | sources and different receivers. And they gonna have the same image point which |
|
| 46:45 | P waves and flatly um in flatly means the midpoint, if the beds |
|
| 46:54 | dipping, it's still approximately true. um And if the overburden is complex |
|
| 47:02 | of simple, that also is gonna out the image points because you |
|
| 47:07 | uh as if, if there's a velocity anomaly and the overburden that's gonna |
|
| 47:14 | the seismic rays as they go through that uh they, they don't all |
|
| 47:19 | up where you thought they were gonna up. So, uh uh that's |
|
| 47:24 | example of overburden complexity which uh affects quality of the image. OK. |
|
| 47:36 | , um the analysis of resolution is gonna depend upon how closely we |
|
| 47:43 | Normally we sample every four milliseconds. But uh maybe in some cases, |
|
| 47:50 | it should be less or some it should be more Uh That is |
|
| 47:54 | design consideration. Uh Normally whoever is the, the uh the survey can |
|
| 48:02 | , I want uh uh uh digital uh every two milliseconds or every 2.5 |
|
| 48:09 | or whatever. Uh Not the uh four milliseconds. Uh The equipment is |
|
| 48:16 | of that. Uh So it's, a conscious decision that he has to |
|
| 48:21 | . And uh uh uh every company its uh own acquisition experts who make |
|
| 48:28 | decisions. And normally they, they spend too much time thinking about time |
|
| 48:33 | . Normally, they spend most of time thinking about space sampling because that |
|
| 48:39 | uh that can be expensive if I that I wanna have space SAMP sampling |
|
| 48:45 | space with AAA tight spacing that's gonna me money. And II I decide |
|
| 48:52 | can be uh a coarser spacing and more space between adjacent receivers that's gonna |
|
| 49:00 | me money. But I don't wanna too much money. I don't wanna |
|
| 49:04 | put the receivers too far apart because uh when, if I put them |
|
| 49:10 | far apart, maybe the data will the question I have is uh where |
|
| 49:14 | oil is. And so, uh the most expensive data is the one |
|
| 49:22 | you uh go out and come back uh data, which is sufficient to |
|
| 49:29 | your question. So that's the most . So if you try to save |
|
| 49:34 | and, and do a survey, is half, half as expensive, |
|
| 49:38 | , it might turn out that that completely wasted. You should have spent |
|
| 49:41 | money to do it properly in the place. So every company has its |
|
| 49:47 | experts who are making these decisions and getting uh it's, it's more and |
|
| 49:54 | complicated as we have more and more options on the ground. So, |
|
| 50:03 | uh finally, uh it also depends the amount of uh signal versus the |
|
| 50:08 | of noise. And so we're just discuss here some elementary points. |
|
| 50:13 | first with vertical resolution and uh uh gonna be different from um uh horizontal |
|
| 50:20 | . So let's talk about the vertical first. So uh uh we said |
|
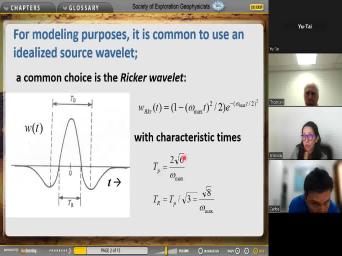
| 50:28 | depends on the wavelength. So let uh uh do this simple analysis using |
|
| 50:33 | ricer wavelength and Arica wavelength has uh uh and remind the amplitude this is |
|
| 50:40 | , but it, it has a shape uh which is determined by this |
|
| 50:47 | only. That's the only um uh parameter in the definition of the uh |
|
| 50:57 | wavel, these two separations between uh uh the two troughs and the 20 |
|
| 51:05 | those depend upon Omega ma. So are the characteristic time you see Omega |
|
| 51:12 | is in here for that's the, , the distance between the two |
|
| 51:17 | And the um uh this distance here uh given by a, a SIM |
|
| 51:24 | has a simple relationship with uh uh this uh delay right here. Um |
|
| 51:34 | hold on. So here's the expression that, this is what the, |
|
| 51:43 | spectrum of like. So as a as a function of uh frequency, |
|
| 51:49 | amplitude uh uh four is is gonna the maximum light here. So |
|
| 51:55 | that's the uh the omega max that talked about the phone. And uh |
|
| 52:01 | is only the real part of the a spectrum. So the, the |
|
| 52:06 | part is uh uh zero. So call this zero phase and that's what |
|
| 52:12 | this thing to be symmetrical. So the real world, we never have |
|
| 52:19 | wavelets as we acquire them because uh wave uh uh uh oh we, |
|
| 52:29 | call this a non causal wave whenever cause a wave, it always turns |
|
| 52:35 | to be front loaded. And this is symmetrical. So the leading trough |
|
| 52:45 | exactly the same as the trailing So this one is symmetrical, real |
|
| 52:50 | is not like that, but we transform real data into a zero phase |
|
| 52:57 | like this for uh uh the convenience the interpreters because the interpreters want to |
|
| 53:03 | about uh when they see a uh a wavelength arriving with a strong |
|
| 53:09 | They want to think that is the the time of the reflector. |
|
| 53:15 | they don't want to mess around with uh asymmetric wavel. So mostly we |
|
| 53:21 | our data for into zero phase data the convenience of the interpreter. So |
|
| 53:34 | means it's a, it's non causal but useful for the, for the |
|
| 53:42 | . Now, if we have just single planar interface, like like we |
|
| 53:48 | um uh in lecture six, then of these frequencies that is all of |
|
| 53:53 | frequencies here, they reflect independently. so the reflected wave has the same |
|
| 53:59 | as the instant wave. Uh So is why we get away with the |
|
| 54:05 | wave reflection analysis. I'm sure you uh when we were talking about this |
|
| 54:11 | uh with our, our plane our reflection analysis with an infinite plane |
|
| 54:17 | . I'm sure you thought uh this right. We have the uh the |
|
| 54:22 | wave coming in and it goes on . Our data isn't like that. |
|
| 54:28 | data is waveless uh localized in Well, we got away with it |
|
| 54:34 | that reflection lecture because all of these be superposed after the analysis. So |
|
| 54:42 | uh uh the reflected wavelength has the shape, same spectrum as the instant |
|
| 54:47 | . So we only have to do one, we have to do one |
|
| 54:54 | at a time. And in that , we found out that the answer |
|
| 54:58 | not depend upon frequency. Very good means the reflection has the same phase |
|
| 55:03 | the in secondary. However, in real earth, there will be other |
|
| 55:09 | nearby. We didn't have a single planar interface with two has space, |
|
| 55:13 | don't have that in the real. uh when these uh other reflectors uh |
|
| 55:19 | their reflections, they're gonna superpose on , on the one that we're interested |
|
| 55:24 | . And that's so that raises new . So here's an example of what |
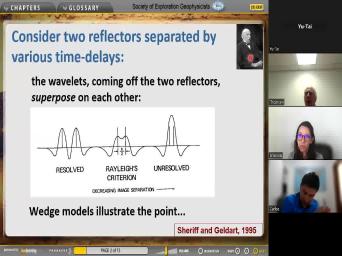
|
| 55:28 | mean by resolution. So this was due to the same guy that's sort |
|
| 55:33 | pompous. This is Lord Raley. would be pompous too if you were |
|
| 55:38 | member of the House of Lords. these days, uh there are few |
|
| 55:43 | any scientists and uh uh uh the of Lords in England, uh I |
|
| 55:52 | know one member of the House of in England, that is uh Lord |
|
| 55:57 | Brown who used to be my Uh He's still, he, he |
|
| 56:03 | , no lo no longer my boss longer works for BP, but he |
|
| 56:07 | still a member of the House of . And uh he was a scientist |
|
| 56:12 | a sort, he, he had bachelor's degree in physics. Um And |
|
| 56:18 | uh uh he was reasonably knowledgeable for manager, he was reasonably knowledgeable about |
|
| 56:24 | business. Um Some managers, you , have uh uh their training in |
|
| 56:30 | accounting or in law, something like . Uh But it's useful to have |
|
| 56:36 | boss who has AAA and some of training in the science, which is |
|
| 56:42 | to the business that he's managing. uh if uh uh Lord Brown had |
|
| 56:50 | in chemistry, I think that might been uh useful for him because we |
|
| 56:56 | a lot of chemistry in an oil , you know, the refineries and |
|
| 56:59 | on. But the exploration part needs background in physics which Lord Brown |
|
| 57:06 | So back in the day, Lord was a member of the House of |
|
| 57:10 | and uh and he was doing his at the same time, he wasn't |
|
| 57:14 | by government agencies, he was supported um um uh oil companies. He |
|
| 57:24 | or he was a rich guy to with. And so in, in |
|
| 57:28 | spare time, he thought about these of wave propagation. So he gave |
|
| 57:33 | early waves and he gave us this of resolution. So imagine two |
|
| 57:39 | Uh uh uh uh uh So here two uh ricker wavelength separated in time |
|
| 57:48 | uh two reflections separated. Here. can see if, if the wave |
|
| 57:52 | impulsive, we would see this one this one it's not impulsive, it's |
|
| 57:57 | a ricker wavelength uh uh uh coming of each one of these reflections but |
|
| 58:02 | far enough apart so that, uh, they're well separated and you |
|
| 58:07 | tell if, if, you know , that this is a ricker wavelength |
|
| 58:11 | only one peak to it you can , ok, we got two, |
|
| 58:16 | here over here. Those events uh, uh, close together and |
|
| 58:22 | we see one event and, uh, you, uh, you |
|
| 58:26 | say, well, uh, I think this is a RW coming |
|
| 58:32 | of a single reflector, but I'm sure it could be two or seven |
|
| 58:37 | whatever. Anyway, whatever they they're closely resolved, uh they're closely |
|
| 58:42 | . So they, we can't resolve separation between these two and in |
|
| 58:47 | you see, uh for separation in um um they are uh partly |
|
| 58:55 | If you, if you saw this a reflected data set, you'd |
|
| 58:59 | OK, probably we have two events there separated. And what is this |
|
| 59:04 | ? This is half of a So let's look at some wedge models |
|
| 59:11 | illustrate the point. So down we have a wedge before we talk |
|
| 59:15 | that. Uh let's talk about this up here. And so there is |
|
| 59:19 | isolated reflector uh giving up um um zero offset reflection um everywhere along |
|
| 59:30 | zero offset reflection. And uh so what is below this? Well, |
|
| 59:38 | can see here is um uh an impeding spot. So we have |
|
| 59:43 | uh uh in the upper half space have impedance Z zero. And then |
|
| 59:49 | have uh uh in between here, have a little bit higher Z we |
|
| 59:53 | it Z plus. And then uh down here and we can see in |
|
| 59:58 | lines, the outlines of a wedge a point right here and a sticker |
|
| 60:03 | here, all that ZW for wedge down here, Z minus uh from |
|
| 60:09 | lower half space. So, with description of the model, let's look |
|
| 60:14 | the uh uh normal trace coming in the fat end of the wedge, |
|
| 60:21 | can see the uh first one coming and the second one coming in very |
|
| 60:25 | that uh that you can see there's top and the bottom to that |
|
| 60:29 | But then look at what happens as go up in here, somewhere in |
|
| 60:35 | , we lose the resolution some maybe about in here, we lose their |
|
| 60:43 | and we can't tell whether this event from uh a wedge or from a |
|
| 60:49 | interface like that. Notice that the is less and uh here, but |
|
| 60:56 | grows, the amplitude is growing And uh what's happening here is that |
|
| 61:04 | , the uh the peak of this is uh is superposed on, on |
|
| 61:09 | . Let me say it the other around this peak is always coming at |
|
| 61:13 | same time as we go to a thinner wedge uh on the, |
|
| 61:19 | the bottom of it, it's closer closer And by this time they are |
|
| 61:24 | exactly on top of each other. so, uh you think, |
|
| 61:29 | so maybe I can uh compute the of the wedge by measuring the amount |
|
| 61:38 | uh the increase here. That's uh uh a possibility. But it, |
|
| 61:44 | uh might be a little bit dangerous you're assuming, you know, uh |
|
| 61:48 | is perfect and, and one like at the bottom of uh or, |
|
| 61:54 | nothing inside the wedge, just a inside the wedge. So if you're |
|
| 61:59 | compute the wedge, the thickness of wedge in this amplitude, um it's |
|
| 62:05 | be um in involving additional assumptions. , let's think about what happens when |
|
| 62:13 | have this situation where you have uh the lower half space has the same |
|
| 62:19 | as the upper half space. So uh which uh I should not the |
|
| 62:25 | half layer, but the uh this not the upper half, this is |
|
| 62:28 | the upper half space. This is the upper half space. This is |
|
| 62:32 | coarse layer impedes that in here. so, uh and we step up |
|
| 62:38 | impedance and then we step back So that means that the bottom reflection |
|
| 62:43 | the opposite polarity than the top And you can see that clearly here |
|
| 62:49 | in between, you can uh the gets lost. So you can't tell |
|
| 62:54 | what you have here and then what's , you see as we get closer |
|
| 62:58 | closer uh we eventually get to have to uh no reflection at all. |
|
| 63:11 | so you see if the thickness of is zero and you have this Z |
|
| 63:15 | uh Z plus all the way down , that's what gives you uniform half |
|
| 63:20 | lower half space and no reflection at . So, yeah, so um |
|
| 63:38 | think about what that implies for the problem. So here's a model of |
|
| 63:44 | reflection problem. Here's our primary, here is uh uh uh uh another |
|
| 63:51 | coming off the bottom of uh this . And if the two are well |
|
| 63:56 | from our problem, if you want didn't see the other ones, but |
|
| 63:59 | they aren't closely separated, they superpose each other. So that's what we |
|
| 64:04 | just talking about. Now, in to that, we might have |
|
| 64:12 | So this is what we call a peg leg multiple. This is |
|
| 64:17 | this is not a peg, this another primary measure, see, but |
|
| 64:21 | in addition, we might have a leg multiple if the layer is very |
|
| 64:26 | , uh this one is gonna superpose these two and with a little delay |
|
| 64:32 | uh uh affect the amplitude and the . And also there's gonna be mode |
|
| 64:38 | see we have here uh uh converted shear wave and con and sheer wave |
|
| 64:43 | back and uh uh coming up and in the cartoon, it converts back |
|
| 64:49 | peak. So uh if it didn't back to P and went on up |
|
| 64:53 | a sheer wave, then it would delayed by uh uh the, the |
|
| 64:59 | to S delay all the way up the overberg. But if it's only |
|
| 65:03 | sheer wave in this, an it's a small delay and uh uh |
|
| 65:08 | local mode conversions and all of these events get uh uh superposed on each |
|
| 65:16 | . And uh if you think of in terms of uh of uh uh |
|
| 65:23 | spikes of arrivals involved with the then uh uh uh that way if |
|
| 65:29 | wavelet has a, depending on the of the wavel, it might, |
|
| 65:34 | could smear all of these together. , um now when you're look at |
|
| 65:43 | real data, these effects are in , but you're looking at it using |
|
| 65:51 | that was written by somebody May Hanson , maybe Hugo, who knows uh |
|
| 65:56 | your favorite uh uh uh package of is. Maybe it's written by uh |
|
| 66:05 | your experts in house. Let me Rosa uh in uh in some, |
|
| 66:10 | the Western G code. Do you internal software or do you use uh |
|
| 66:16 | , it's internal pare only. Yeah. Yeah. From patrol. |
|
| 66:21 | you need to uh whenever you're using , you want to uh uh talk |
|
| 66:26 | the real experts. You, you're gonna be a, a deep uh |
|
| 66:31 | , you're a broad expert, you're interpreter you, you concern yourself |
|
| 66:35 | So many things that a deep expert know anything about, but he knows |
|
| 66:41 | what's the details of the software. you should talk to your patrol experts |
|
| 66:45 | , and ask him uh uh did you include all this stuff in |
|
| 66:50 | or did you include only the Do you know the answer to |
|
| 66:55 | Verda? No. Iii, I know. Yeah. So, so |
|
| 67:05 | , it's very common that um uh interpreter uh uh is gonna take at |
|
| 67:11 | value whatever uh software is given to or to him, you shouldn't do |
|
| 67:17 | . You should be skeptical and you ask the experts who know the details |
|
| 67:22 | that software. Well, did you about this? Did you think about |
|
| 67:26 | ? And uh oh, it's possible guy says uh uh well, you |
|
| 67:33 | , I don't know, I didn't about that. I didn't write this |
|
| 67:36 | of it. Let me go speak my other colleague who's in the other |
|
| 67:40 | at the other end of the hallway we'll come back with you for an |
|
| 67:44 | . You should be uh uh uh uh uh Rosa, you should be |
|
| 67:49 | difficult customer for your internal experts in trial. And so from your um |
|
| 67:57 | questions, they will improve the you know, uh uh they, |
|
| 68:02 | might say, gosh, we didn't about that either. Uh We are |
|
| 68:05 | go back and uh you know, I improve the software and uh give |
|
| 68:11 | a new, we'll give you a version of the patrol software um in |
|
| 68:16 | months time and it will have the version, um by default. |
|
| 68:21 | and then you can click the button also include these sorts of issues |
|
| 68:27 | and decide for yourself whether it makes difference or not. That's the way |
|
| 68:32 | should happen. And they should appreciate , um, your, uh you're |
|
| 68:39 | questions. Of course, you don't them in a way you're speaking with |
|
| 68:44 | . Uh But uh you should ask and by the way, uh for |
|
| 68:50 | that uh the same that could go uh people here at the university, |
|
| 68:56 | we use a lot of third party . We use Patrol, we also |
|
| 69:01 | Hanson Russell and uh uh we should asking ourselves these questions and if we |
|
| 69:08 | get good answers, we ought to up the uh people behind the software |
|
| 69:12 | ask them and um usually they appreciate . They say, oh, |
|
| 69:17 | we thought about that but uh uh thought it wasn't important. So we |
|
| 69:21 | include it. Uh So at that , you said, well, what |
|
| 69:26 | you think it's not important, don't think you should include it? And |
|
| 69:29 | look to see whether or not it's and you might find that it's important |
|
| 69:34 | some cases, not important in but it's a good idea to uh |
|
| 69:39 | include um everything you can think of uh uh for uh simple cases, |
|
| 69:50 | maybe it's the, the complications are important, but the way you find |
|
| 69:55 | is by including it and offering it an option to the users. And |
|
| 70:03 | , uh uh my favorite example, this is one of my favorite examples |
|
| 70:07 | here. Another one of my favorite is anisotropy. Should we uh be |
|
| 70:13 | anisotropy in our a discussion of these as they vary with offset, you |
|
| 70:21 | , as they vary with offset, the same as varying with angle of |
|
| 70:26 | . And so uh uh we should asking ourselves, does it make sense |
|
| 70:31 | analyze amplitudes as a function of angle we're ignoring velocity as a functional angle |
|
| 70:40 | the velocity of the functional angle? That's an isotropy approach. So the |
|
| 70:45 | to that uh uh is that definitely should include an isotropy and we'll talk |
|
| 70:51 | that more next Friday. So how horizontal resolution now? Uh because the |
|
| 71:04 | zone is so large, you would you, you, you could confirm |
|
| 71:09 | resolution is the worst because you get smearing from all over, from the |
|
| 71:15 | from the fren zone effects. So you have a AAA wedge, like |
|
| 71:21 | showed uh with the fractions and uh uh around 100%. Uh Well, |
|
| 71:33 | that, that, that picture uh the wedge and the diffraction is not |
|
| 71:38 | guru, but you can imagine in subsurface, there will be lateral heter |
|
| 71:44 | , you know, like river varied river channels in the subsurface. |
|
| 71:48 | you want to be able to resolve . And you would think from everything |
|
| 71:52 | said so far that uh we can't those because we're gonna get smearing from |
|
| 71:58 | over this for uh zone. And uh the answer is that uh uh |
|
| 72:07 | , uh it's not a problem we have better resolution horizontally, |
|
| 72:13 | Uh uh But it does depend upon acquisition design. And um it also |
|
| 72:21 | uh depends, normally have a acquisition with lots of receivers on the |
|
| 72:29 | And then we do imaging with And since we have so much |
|
| 72:34 | so many uh sources and receivers going our migration algorithms, uh uh Normally |
|
| 72:42 | uh overcome the fel zone effects uh and we get amazing resolution uh uh |
|
| 72:51 | with modern uh methods of data processing uh including uh coherency processing. And |
|
| 72:58 | are uh there are many variants of and there's uh my, my favorite |
|
| 73:04 | called spectral decomposition. And when you at these migrated uh uh images with |
|
| 73:12 | processing or spectral decomposition, you find reflection. So this is an example |
|
| 73:19 | of a Buried river system from my uh colleague uh uh Greg Parti about |
|
| 73:27 | years ago. And this looks like air filter, doesn't it? But |
|
| 73:32 | river is uh many millions of years . And uh today there is no |
|
| 73:38 | at that point, there's something else , this was 10,000 ft down and |
|
| 73:43 | can see everything you can see all tributaries. You can see the uh |
|
| 73:47 | how it's different on the, the side of the curve and the outside |
|
| 73:51 | the curve. So many uh details can see here and just the fact |
|
| 73:55 | it looks so geologic, that means is not noise we're making, this |
|
| 74:01 | signal. And so I will uh talk about how they do this very |
|
| 74:07 | techniques about how they do this. it's just amazing horizontal resolution we get |
|
| 74:13 | modern methods of acquisition and process. uh let's have a couple of quick |
|
| 74:21 | here. We're almost done with the . We are done with the |
|
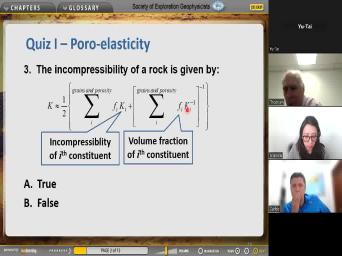
| 74:25 | Uh um So I uh is this or false? Let me ask uh |
|
| 74:31 | , is this uh uh um So that's a good uh uh verbal |
|
| 74:38 | of what we mean by resolution. uh Carlos, how about you says |
|
| 74:44 | estimation of resolution in real data requires the wavelet be reshaped to a ricer |
|
| 74:51 | . Is this true or false? ? I wanna hear you thinking out |
|
| 75:09 | . Sorry because uh yeah, I , I was, I was saying |
|
| 75:12 | I think it's false. Yeah, false. It uh it's often done |
|
| 75:16 | the record wave but we could uh could shape what we do is we |
|
| 75:21 | techniques uh which, which Professor J tell you about how we can shape |
|
| 75:26 | wavelength of the real data into anything want. Well, not anything we |
|
| 75:31 | . We can't shape it into perfect . But if we uh uh uh |
|
| 75:36 | can, we have an, we uh we can shape it to wavelengths |
|
| 75:41 | are sharper wavelengths uh uh uh than present in the raw data by clever |
|
| 75:50 | which he'll tell you about. Uh uh we could uh be shaping it |
|
| 75:54 | a ricer wave or we could shape to a zero phase wavelength which is |
|
| 75:59 | a ricer wavel. You know, all it means is Syme zero phase |
|
| 76:04 | symmetric. Uh And it refers to uh the uh the imaginary part of |
|
| 76:11 | fair spoke spectrum is uh zero. this is false. Uh It's too |
|
| 76:22 | . Um uh uh Rosa uh And how about this one true or false |
|
| 76:30 | beds are well reserved vertically. And two way travel time between the top |
|
| 76:35 | bottom reflection is greater than half of dominant um period. Is that true |
|
| 76:42 | false? They're well resolved when the time difference is greater than half the |
|
| 76:53 | period. I think it's true. . In fact, that was the |
|
| 76:58 | the criterion that Lord Raley proposed. so you could propose another one, |
|
| 77:03 | let's go with the Lord really, mean he was a member of the |
|
| 77:07 | of Lords. So it must be . Right. Uh Well, uh |
|
| 77:11 | this is sort of AAA conventional understanding what we mean by vertical resolution. |
|
| 77:18 | , back to you Lily there, the horizontal resolution is limited by the |
|
| 77:24 | for the for Rel zone, which saw back on page 58. And |
|
| 77:30 | in that formula that the depth uh is included in the size of the |
|
| 77:37 | forel zone. Uh So the Chanel can be quite uh large at great |
|
| 77:43 | . Is, does that mean that resolution is quite poor and right and |
|
| 77:48 | there? Oh They may, I hear you thinking out loud. |
|
| 78:01 | And uh and your voice is very . So, uh yeah. |
|
| 78:06 | that is uh so uh that we about that uh earlier. Uh This |
|
| 78:17 | exactly what, what you would think , when we discussed for ozone, |
|
| 78:23 | know that you were thinking, what that means is that uh uh |
|
| 78:27 | gonna smear events uh horizontally together and not gonna have good horizontal resolution. |
|
| 78:33 | in fact, uh uh we talked five minutes ago, we talked about |
|
| 78:37 | modern methods of wave propagation with uh sources and many receivers and clever processing |
|
| 78:47 | those F forel zone effects. actually, we have uh uh uh |
|
| 78:52 | , it's correct. Uh uh So , this statement is false because of |
|
| 78:59 | techniques overcome uh the objections of Mr . So um the next topic is |
|
| 79:09 | reflector. But since we talked about , uh, before, uh, |
|
| 79:15 | , I'm gonna skip over this. , we're a bit behind time |
|
| 79:19 | So I'm gonna skip through this business curve reflectors and you remember this figure |
|
| 79:25 | before? And so, uh, , I'm gonna skip over that |
|
| 79:33 | um, I think, I think want to skip over this whole part |
|
| 79:47 | her reflectors. You'll see it in lectures, uh, which are, |
|
| 79:53 | , in on canvas. But because on time and because we're behind |
|
| 79:59 | I think I want to skip No, I uh let's see |
|
| 80:16 | Yeah, I want, I want skip over uh this party. So |
|
| 80:21 | , I will guarantee you, you're gonna be tested on this, about |
|
| 80:25 | things. And so it's a little complicated and so I'm gonna just skip |
|
| 80:31 | it. A lot of interesting stuff there, isn't there? You could |
|
| 80:39 | an interesting picture so that uh uh brings us to the end of this |
|
| 80:45 | of complications. And this is what would call the classical program of uh |
|
| 80:51 | and waves. But there are other as we went through these, we |
|
| 80:56 | certain assumptions which are not true. so now let's go back and think |
|
| 81:03 | what are the uh implications of And so there are three topics |
|
| 81:09 | uh uh poor elasticity, attenuation and . So let me just review these |
|
| 81:15 | uh poor elasticity happens because we we want to apply this thinking to |
|
| 81:23 | and rocks have grains and pores. that was explicitly excluded back here when |
|
| 81:29 | talked about Hook's Law, Hook's Law that the medium is uniform like glass |
|
| 81:36 | like copper or steel, not like . And so we um um uh |
|
| 81:44 | naively applied Cook's Law to do all understanding in here, knowing that the |
|
| 81:51 | day, we made approximations, we assumptions which are wrong. And now |
|
| 81:56 | gonna go back and talk about uh propagation of waves and real raw. |
|
| 82:04 | . And then there's another assumption we here shortly after we made the Hoola |
|
| 82:09 | . Well, maybe at the same , we realized that Hola does not |
|
| 82:14 | for attenuation. And uh ation is our data. Whenever we look at |
|
| 82:20 | data set, we look at uh uh arrivals coming in at long |
|
| 82:26 | those have lower frequency content than the at short times. So that means |
|
| 82:34 | the, the wave has lost high as it propagates that's due to attenuation |
|
| 82:40 | surely that must be important. So correct. It is important, but |
|
| 82:45 | ignored it all throughout here. And we're gonna take it up in chapter |
|
| 82:51 | . And then uh uh we're gonna uh both of these today. And |
|
| 82:56 | uh next Friday, we're gonna talk another simplifying assumption that we made. |
|
| 83:03 | back here. We assume the rocks isotropic when uh uh we know just |
|
| 83:10 | looking at them, they must be isotropic. So those three assumptions that |
|
| 83:18 | made obviously wrong. We did we made it anyway to get |
|
| 83:23 | uh, uh initial understanding and now gonna go back and, uh, |
|
| 83:30 | what happens when we drop those limiting . So, what I'm gonna do |
|
| 83:36 | is to, uh, uh, , she, yeah, that ends |
|
| 83:45 | , the slide show and now I'm to bring up the next topic. |
|
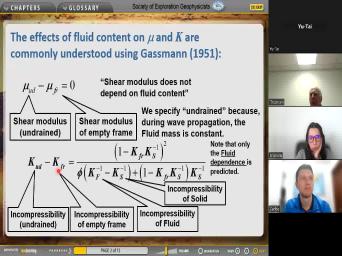
| 83:56 | No. And I think you, , I, I think, |
|
| 84:05 | are you looking at the, at beginning of poor elasticity? So |
|
| 84:10 | you see this slide? Yes. . OK. OK. OK. |
|
| 84:14 | because of the way I did it came up automatically. So, |
|
| 84:23 | , here's our objectives for this, for this lecture, we got to |
|
| 84:31 | Hook's Law. Hh Was a great , but he lived a long time |
|
| 84:37 | and he made unrealistic assumptions. I, I think it was good |
|
| 84:40 | for him. It solved the problem was in front of him at that |
|
| 84:45 | , but here we are 300 years and we're trying to apply those |
|
| 84:50 | real walks. So we've got to modify his assumption. No. Um |
|
| 85:05 | we do that, when, when consider real rock, we're gonna uh |
|
| 85:12 | there's gonna be a pressure dependence and gonna depend upon something we call effective |
|
| 85:19 | . So that's not an easy uh . So we'll spend some time on |
|
| 85:27 | . And um then uh uh we're see that the, the velocities of |
|
| 85:34 | rocks depend upon what's, what is fluid in the pore space. I |
|
| 85:45 | you probably already know uh uh uh answer to this question. Uh |
|
| 85:52 | almost everybody in our business understands the of fluids on rock philosophies following uh |
|
| 86:02 | done by uh uh by uh the in 1941 before any of us were |
|
| 86:09 | , even before I was born and by Gasman in 1951 uh 10 years |
|
| 86:17 | . And uh basically, our standard is uh is unchanged for all that |
|
| 86:24 | , almost three quarters of a but it's recently been discovered that that |
|
| 86:30 | by Gasman is wrong. And so gonna talk about that. Finally, |
|
| 86:40 | at high frequencies, uh we're gonna a new type of weight, a |
|
| 86:46 | type of wave uh because we're dealing heterogeneous rocks, not homogeneous solids. |
|
| 86:53 | have a new type of wave. about that? So everything we've done |
|
| 87:00 | far has been classic seismology equally suitable exploration or for the understanding the deep |
|
| 87:07 | of the earth, but none of is truly suitable for exploration since it |
|
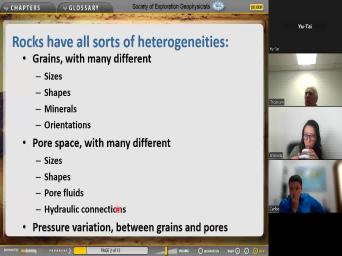
| 87:13 | the effect of ferocity and because of poorest, that's what's paying our salaries |
|
| 87:19 | of the foods in the poorest. rocks have all sorts of heterogeneity, |
|
| 87:24 | example, uh grains and pores talk the grains, the grains have many |
|
| 87:29 | sizes, shapes, minerals and So every one of those minerals in |
|
| 87:36 | rock is an isotropic but uh uh let's assume for now that they're randomly |
|
| 87:46 | so that the uh the anti averages . And so we can uh uh |
|
| 87:52 | uh regard any the rock as a but an isotropic with grains and |
|
| 88:04 | And then the pore space. How the pore space? It's got a |
|
| 88:08 | complicated pore space. And so parts it, uh uh uh uh we |
|
| 88:13 | think of uh depending on the shape the grain, the pores might well |
|
| 88:19 | um uh described as uh uh you , uh uh spherical pores. If |
|
| 88:29 | , if you had the grains to um uh only uh grains of |
|
| 88:35 | only grain of sand. Uh then between the grains of sound are pores |
|
| 88:41 | we uh they're not spherical, but they are more or less the same |
|
| 88:46 | in all directions. So we call excellent forms by contrast, uh uh |
|
| 88:53 | they could have uh the shape of crack, it could have the shape |
|
| 88:58 | a, you know, a we call those penny shaped cracks. |
|
| 89:01 | course, that's too idealized. But clear that uh the, the core |
|
| 89:07 | has um a very complicated shape. of a lot of us is connected |
|
| 89:13 | , hydraulically connected together. Um And like to think we can think about |
|
| 89:18 | throats and more volumes. And so . But those are all idealizations. |
|
| 89:23 | best thing to see uh to say pore space is, has a very |
|
| 89:28 | shape and uh very complicated hydraulic connections the various parts of that part |
|
| 89:36 | And you will see shortly how the connections are go are gonna affect wave |
|
| 89:44 | . Now, also we're gonna learn it depends upon uh um uh what |
|
| 89:49 | are in those pores. And of , it uh that's what I said |
|
| 89:53 | . Uh the hydraulic connections, imagine , that a rock has a complicated |
|
| 89:59 | space as a wave is going it could be squirting the fluid around |
|
| 90:04 | the, the pore space, moving fluid on the grain scale uh between |
|
| 90:09 | different parts of the P space. you can imagine that could be |
|
| 90:13 | And so the extent by which that's depends upon uh the hydraulic connection between |
|
| 90:19 | various parts of the P space. here's something you might not have thought |
|
| 90:25 | . Uh the primary variation. Of , the pressure in the poor space |
|
| 90:33 | is different in the pressure and the . That's true both for as a |
|
| 90:38 | is going through and uh already as uh as the rock is sitting there |
|
| 90:46 | for the grain for the wave to , just think of a rock sitting |
|
| 90:51 | under uh uh thousands of feet of over bird. And uh so uh |
|
| 90:57 | gonna have a weight pushing down from and also from the sides. And |
|
| 91:02 | is it the, the stress coming the sides is not the same as |
|
| 91:07 | stress coming from above? So that to, uh uh uh no, |
|
| 91:16 | leads to an effect on the mechanical of the rock. When the wave |
|
| 91:24 | , when the wave arrives, it's uh be passing through that rock according |
|
| 91:30 | the physical properties of the rock as compression above. And it's as its |
|
| 91:35 | aside. Now, in that same is gonna be four space with |
|
| 91:42 | Let's assume that the fluids are That's normally the case fluid is |
|
| 91:48 | So the uh before the wave gets , the R is gonna have a |
|
| 91:55 | pressure on it than the grains because of the load is carried by the |
|
| 92:02 | . And so the uh uh uh grains are gonna have a smaller |
|
| 92:09 | And furthermore, it's gonna help um be easy to predict in advance. |
|
| 92:16 | uh uh The, the uh the in the grains is pretty easy to |
|
| 92:22 | because that just comes from the weight the over. So you can just |
|
| 92:27 | up all the weight in the over you can figure out it to a |
|
| 92:30 | approximation, what is the state of in the grains? But for the |
|
| 92:36 | , it's different because uh there might uh uh uh uh places in the |
|
| 92:44 | where the uh or the hydraulic connections been help have disappeared over geological |
|
| 92:55 | There could be seals in the overburden prevent the fluids from moving around over |
|
| 93:04 | time. As a matter of that's good for us because some of |
|
| 93:08 | seals confined oil reservoirs. Most of don't. Of course, most of |
|
| 93:15 | , most of those seals can find reservoirs. And normally we're not interested |
|
| 93:20 | the brine, but just the fact we have permeability barriers in the subsurface |
|
| 93:26 | that uh uh the uh the foods move around uh everywhere because they're are |
|
| 93:35 | by these seals. Seals are not in the rock, but uh and |
|
| 93:41 | some places in the rock, both seals and uh vertical seal. So |
|
| 93:47 | fluid is not free to move around whatever. And so that means that |
|
| 93:53 | look, yeah, beneath that you don't know what the pressure in |
|
| 93:58 | food could be. You do know it's less than the pressure in the |
|
| 94:06 | . But here we have a situation deep inside the earth where the pressure |
|
| 94:11 | the fluid pressure in the grain is high value of pressure. And right |
|
| 94:16 | to it just uh uh uh a away is a, a brine with |
|
| 94:23 | , with a very different pressure, of of, of p si thousands |
|
| 94:29 | pounds per difference in the pressure between , ok, the pressure in the |
|
| 94:38 | and the pressure in the food. just think what that is gonna do |
|
| 94:42 | the mechanical properties of the rock and gonna affect the, what happens when |
|
| 94:48 | wave comes through. OK. So start this one at a time. |
|
| 94:54 | lot of complexity here we have to with. So first, let's handle |
|
| 94:58 | mineral heterogeneity. Uh uh because we that most rocks have many different minerals |
|
| 95:04 | them. And so, uh uh , the, the, the density |
|
| 95:12 | the rock is gonna be uh simply a weighted average of the density of |
|
| 95:19 | different minerals. So we have here we have a sum over all the |
|
| 95:23 | . Um and we have um a density for each mineral density for |
|
| 95:31 | , the density for calcite, a for pla like all those minerals have |
|
| 95:36 | densities, usually between two and 3 per cubic centimeter. Uh There might |
|
| 95:42 | some very heavy gras in there but they will have small volume fractions. |
|
| 95:48 | uh the way we uh think about is we realize that the density of |
|
| 95:53 | uh uh on the rock is this , an average of all those mineral |
|
| 95:59 | . So there is the notation. this uh operation of just adding up |
|
| 96:05 | the mass and a volume. That's leads to this equate. So if |
|
| 96:20 | have a sample of rock in, your hand, in the laboratory, |
|
| 96:24 | can uh look at it under an optical microscope or uh maybe as |
|
| 96:31 | , we don't know how to do . But we have friends who are |
|
| 96:34 | and in particular, some of those are photographers. So they're experts in |
|
| 96:41 | . So they can look uh at sample uh through special microscopes and they |
|
| 96:46 | determine all the minerals that are there the fractions of each. And uh |
|
| 96:51 | this is basically, basically a solved . We know we need more than |
|
| 97:00 | oh by density we need. So think about uh an isotropic rock where |
|
| 97:10 | uh the stiffness modulus, that's the we need. And that's what we |
|
| 97:17 | it M and that we know that's to K plus four thirds me. |
|
| 97:21 | let's think about the K part separately the new part. And let's try |
|
| 97:25 | same idea uh for the in compressibility we did for the density. This |
|
| 97:30 | was first had by a guy named , one of these German physicists uh |
|
| 97:35 | in the last century. Uh uh uh rotation is uh very similar to |
|
| 97:42 | we had before. However, when do this, it just means that |
|
| 97:47 | added up all the in compressibility. that concept it makes no sense. |
|
| 97:52 | up all the mass makes sense, adding up in compressibility, that just |
|
| 97:57 | make any sense. What does that ? So uh we need to have |
|
| 98:00 | better analysis. And so um what did was he derived this uh uh |
|
| 98:07 | expression and the way he derived it he made the uh the assumption that |
|
| 98:12 | strain is uniform in all the But of course, that's unrealistic, |
|
| 98:17 | be stiff grains and soft grains. so he, he knew that wasn't |
|
| 98:22 | . But he thought with, by , as an approximation, maybe that's |
|
| 98:27 | enough, maybe it was in his . But that was a long time |
|
| 98:30 | , we need to have better analysis . And so one thing we could |
|
| 98:37 | is we could uh instead assume that the stress is uniform instead of assuming |
|
| 98:43 | strain is uniform. That's uh assume stress is uniform. That assumption was |
|
| 98:49 | by a guy named Roy Roy. you see how I'm pronouncing that German |
|
| 98:54 | , it's not Ruth, it's Roy in German. And at least to |
|
| 98:59 | following formula would simply have a uh volumetric average volume weighted average of the |
|
| 99:08 | of the in compressibility. So that is we, we call that the |
|
| 99:14 | of the uh uh of the And if we uh what I just |
|
| 99:21 | you here is that uh this one actually uh a valid formula. If |
|
| 99:25 | have mixtures of fluids like um uh oil and gas and water, we |
|
| 99:31 | use a formula like this for the the in compressibility of those fluids, |
|
| 99:38 | it doesn't work for grains because for , this assumption is not realistic. |
|
| 99:44 | that this was all done maybe uh years ago and uh about 70 years |
|
| 99:50 | . Uh uh uh Another guy came , his name was Hill and he |
|
| 99:56 | that the void average is an upper and the Royce average is the lower |
|
| 100:02 | . So Royce uh suggested that so let's just make an average of |
|
| 100:07 | . And then, so that's called Roy Roy Hill average. But there |
|
| 100:11 | no justification for that at all. , that's OK. That's just uh |
|
| 100:16 | suggestion by help. So let's do same thing with the uh sheer |
|
| 100:23 | Uh Here's the war average of the Module Act, one average of the |
|
| 100:29 | mineral uh Sheer Module Act. Um voice average is the average of the |
|
| 100:36 | of the, of the sheer Uh uh Hill also showed that uh |
|
| 100:42 | are upper and lower limits. So propose we do uh for large hill |
|
| 100:48 | here. And so these suggestions by are frequently followed today, 70 years |
|
| 101:00 | . And the reason is um uh uh the minerals are broadly similar to |
|
| 101:06 | other. So if we, if variation among the different sheer mono |
|
| 101:12 | you know, only a small maybe uh uh uh you know, |
|
| 101:16 | will be um a rigid uh rigid and ones which are not so |
|
| 101:21 | But if the uh if the the sheer marginal I differ by only |
|
| 101:27 | 20% 30% something like that, maybe can get away with a crude approximation |
|
| 101:33 | that. There's no justification it's we just say that, um, |
|
| 101:38 | what we're gonna do because we can't anything better. Baby two couldn't, |
|
| 101:44 | do anything better. But, I'm gonna suggest there are ways to |
|
| 101:48 | better and we'll, uh, see later. Here's one way to do |
|
| 101:55 | . Um, um, yeah, , some years after Hill, these |
|
| 102:03 | , Ashen and Styne, uh, Americans, by the way, |
|
| 102:08 | uh, those that you can uh, uh bounds, uh uh |
|
| 102:13 | are better than the VO and voice . Let's go back here. Uh |
|
| 102:17 | This is an upper limit. This a lower limit. And so, |
|
| 102:21 | , and have found that, that they made uh more assumptions, they |
|
| 102:27 | get tighter bounds. And so, here is an expression for their |
|
| 102:32 | It says that uh uh let's consider case where uh one identifies the, |
|
| 102:39 | mineral. Uh This is uh this the result uh for two minerals on |
|
| 102:45 | rock composed of two minerals. uh the, the sums here, |
|
| 102:51 | sums only go from 1 to And so let's assume that the uh |
|
| 102:56 | the one with the mineral with index , that's the softer of the two |
|
| 103:03 | in, in compressibility and in So the, the what and Stickman |
|
| 103:10 | was that the uh the in compressibility a random mixture of those two minerals |
|
| 103:23 | between these two bounds uh uh this and this bound. And so |
|
| 103:30 | the two bounds are, uh you , uh this is the soft |
|
| 103:36 | Uh And uh you see in the, the volume fract, various |
|
| 103:39 | fractions. And you can see uh uh this also depends upon the |
|
| 103:46 | matras of the soft rock. And is the sheer modulus of the hard |
|
| 103:52 | . And that's, that all goes to make um oh uh the lower |
|
| 104:00 | and here is the upper route also and you have similar things uh uh |
|
| 104:08 | small for that and sheer Mars and actions shipment gave us formula for doing |
|
| 104:22 | , many men but o only for minerals. Um uh And you can |
|
| 104:30 | , you can imagine if you have minerals, it's gonna be more complicated |
|
| 104:36 | um many geophysics, I would say geophysicist don't apply these, these uh |
|
| 104:43 | these limits are well established theoretically and could do uh uh an average of |
|
| 104:53 | . You would say that OK, I'm gonna assume that the uh |
|
| 104:56 | you know, uh the incompressible of rock is halfway between this lower medium |
|
| 105:03 | this upper medium. Good. Say and how most people, most of |
|
| 105:08 | in our profession don't do that because harder to um apply this theory. |
|
| 105:15 | gotta know the sheer modules as well the uh uh if you wanna get |
|
| 105:22 | bounds on the in compressibility, you to know the sheer margins as well |
|
| 105:27 | both sides. So it's up, rarely done. And one reason it's |
|
| 105:37 | were done is because it also makes assumptions. Those minerals were bound to |
|
| 105:44 | anisotropic. And so I'm not aware anybody um uh making uh uh a |
|
| 105:54 | and isotropic extension of this theory. it's been now 80 years, |
|
| 105:59 | Been more than 80 years. It's , uh uh 60 years, it's |
|
| 106:03 | for some uh uh smart uh uh to say, OK, let's use |
|
| 106:09 | same logic as uh this guy. drop this assumption of isotropy and uh |
|
| 106:15 | what, what it would look like an isotropic mes. OK. Uh |
|
| 106:23 | a good theoretical problem. Wouldn't require experimental work at all, but it |
|
| 106:29 | yet been done. Why hasn't been ? It's because mostly these minerals are |
|
| 106:36 | more or less like each other. willing, really, we should be |
|
| 106:45 | on the differences between the solids and SLS. So you will have noticed |
|
| 106:51 | in the uh equations that we've uh used up until now, the porosity |
|
| 106:57 | not appear we have a K and mu in a row. But uh |
|
| 107:01 | all coming from Hooks analysis. And , I I don't see in those |
|
| 107:09 | anything, the process doesn't appear Now, these ones are implicit functions |
|
| 107:16 | composition, including the compositions of uh the grain, the and the composition |
|
| 107:23 | the pores. Also, you don't in the previous expression, you don't |
|
| 107:29 | any place where the, the pressure . So uh uh we're gonna learn |
|
| 107:37 | these properties are implicit functions of But the pressure on the grounds, |
|
| 107:43 | , on the greens is different than , the pressure on the pores. |
|
| 107:48 | . Isn't it? See how, co as soon as we start talking |
|
| 107:51 | heterogeneity, things get very complicated. , we need to introduce more |
|
| 108:00 | And the reason is that uh the the, the pore filler is so |
|
| 108:05 | from the grain. So if we water in the pores, the water |
|
| 108:09 | an in compressibility which is only about uh 5% of the uh in compressibility |
|
| 108:17 | uh the minerals. And the sheer modules I flirted was zero. |
|
| 108:25 | the, the, the, the in the density is not so |
|
| 108:29 | It is water has the density close one and uh the rentals have density |
|
| 108:35 | two and three. So uh uh is not such a big issue, |
|
| 108:40 | this is a major issue and this also a major issue. So mm |
|
| 108:47 | now, this is really the the first place we've encountered all during |
|
| 108:54 | of course, ever talked about K until this cars time we were talking |
|
| 109:00 | wave propagation. And we use we use the uh the symbol M |
|
| 109:06 | describe the stiffness of the rock in wave propagation. And we knew that |
|
| 109:14 | equals K plus four thirds new uh but neither the K but the K |
|
| 109:19 | not appear anywhere in our wave propagation equation except except in these, in |
|
| 109:26 | combination, this one right here, plus four thirds M. So we |
|
| 109:30 | gave it a name, we call M and we did all of our |
|
| 109:34 | in terms of so up until we didn't ever need to men mention |
|
| 109:41 | itself or the, the lame But now that we have uh more |
|
| 109:50 | assumptions and how we're assuming we're gonna what we have to do to deal |
|
| 109:54 | real rocks, modify the previous analysis extend to real rocks, uh uh |
|
| 110:02 | in both the solids and the And uh so, uh right now |
|
| 110:09 | the first time, we need to at a explicitly because they're two |
|
| 110:15 | there's a possibility for them either to to deform together, yeah, separately |
|
| 110:24 | . So imagine a wave going through rock filled with water. So it |
|
| 110:29 | happen that the wave, the water the uh as the P wave comes |
|
| 110:35 | the rock that there are, you , you know, uh atoms in |
|
| 110:43 | water move in the same direction in same phase as the atoms in the |
|
| 110:49 | . That's sort of uh uh Uh But there is the other possibility |
|
| 110:55 | they could, the grain that the atoms in the water could move |
|
| 111:01 | the opposite direction from the atoms in grans. You can see how that's |
|
| 111:06 | theoretical possibility, although it seems a bizarre. Well, it turns out |
|
| 111:12 | that can happen and, um, , and we are going to return |
|
| 111:22 | this topic, um, by the of this lecture. But for now |
|
| 111:31 | , we're gonna consider, uh, , uh, where the, |
|
| 111:35 | the fluids and the solids move in with each other that's gonna make ordinary |
|
| 111:42 | , just like those that we've been for the past, uh, seven |
|
| 111:46 | , uh uh with only minor modifications that, so that should make you |
|
| 111:51 | happy. Uh uh When we are gonna, we're only gonna say a |
|
| 111:57 | words at the end of this lecture this new type of waves where the |
|
| 112:03 | move out of shades with the And I'll tell you why, ordinary |
|
| 112:11 | for it. OK. So I'll a little quiz here. Uh Let |
|
| 112:16 | turn to, um, uh le is this uh uh uh uh uh |
|
| 112:23 | of these do you think here? Let, let me ask you, |
|
| 112:27 | um only about um uh a uh then I'll go to, to, |
|
| 112:34 | Carlos for being shot at. Is true that the theory of elasticity is |
|
| 112:40 | strict, strictly applicable to because they heterogeneous. Is that true? |
|
| 112:46 | that is true. And uh uh about um, a beat is um |
|
| 112:54 | uh Carlos, is it true that theory of elasticity is not strictly strictly |
|
| 112:59 | the rocks because the rocks are I think that is also true. |
|
| 113:05 | . Well, it's true the way did it, we uh we applied |
|
| 113:10 | uh uh uh the theory of elasticity isotropic rocks. But we're gonna uh |
|
| 113:15 | we didn't have to do that. think about uh uh uh crystal of |
|
| 113:20 | crystal of quartz uh is homogeneous and has a, an outer shape uh |
|
| 113:26 | uh shiny faces and so on on outer shape of the of the crystal |
|
| 113:31 | quartz. And so, you know that shape is determined by the arrangement |
|
| 113:36 | atoms on the inside of the And so, naturally, that's gonna |
|
| 113:39 | making uh waves which are traveling with velocities and in different directions because of |
|
| 113:49 | small scale um arrangement of the atoms the quarks. So it is homogeneous |
|
| 113:56 | hook assumed uh but it's gonna be isotropic and in fact, 300 years |
|
| 114:01 | book uh included anisotropic solids in his . So this one is false. |
|
| 114:09 | . Oh So uh turning to you uh uh is this is the theory |
|
| 114:15 | elasticity is not strictly applicable to rocks inside the earth, those rocks are |
|
| 114:21 | to high pressure is, is that fault? I mean the statement is |
|
| 114:27 | , but that is not the reason the Yeah. Yeah. Yeah, |
|
| 114:33 | , that's correct. Uh So uh could have uh uh solids. So |
|
| 114:39 | to the high pressure and hook would been uh very happy to apply his |
|
| 114:43 | to that. So, uh the one of these uh which is true |
|
| 114:48 | uh a. Mhm. OK. uh the next one, so uh |
|
| 114:54 | got back to you uh Lily. Is this true or false? |
|
| 115:01 | that's true. That's just what we before. Uh So for you |
|
| 115:06 | uh is this uh uh true or ? You too? OK. Just |
|
| 115:33 | you will recognize here, uh uh you, you will recognize this formula |
|
| 115:43 | uh uh uh pretty much like the that we had for uh voice averaging |
|
| 115:49 | the in compressibility. And this looks voice averaging of the in compressibility. |
|
| 115:54 | so we're summing those two sums together probably should have a, a square |
|
| 116:01 | ring around this up and then we're by two. So this looks like |
|
| 116:07 | Vo Roy Hill averaging of the VO and the voice average. But |
|
| 116:12 | it, it includes, it includes , the grains and the pork whereas |
|
| 116:18 | only included the grain. So, um what do you think? Is |
|
| 116:23 | true or false? Yeah, I think it's true. Uh |
|
| 116:29 | I'm gonna say it's false. I'm say it's false. Uh uh uh |
|
| 116:34 | uh uh number one making this average the, of uh this average average |
|
| 116:41 | this average with a half hour Uh That's just a suggestion. And |
|
| 116:46 | also, um we really uh we use that as an approximation, but |
|
| 116:52 | have to recognize it as an Uh uh But the main thing I'm |
|
| 116:57 | about here is that uh um it's in here uh the, the grains |
|
| 117:04 | the core. And so we never uh uh Phil uh Ne and Voight |
|
| 117:11 | included the pores in that sun. back over the um uh in previous |
|
| 117:16 | slides. And you'll see, we include the pores in, in |
|
| 117:20 | Uh So that makes a problem. And, and, and it's not |
|
| 117:25 | it's, it's not very um um , a good idea. Uh oh |
|
| 117:31 | , that's another approximation, include the in here and think about this |
|
| 117:36 | think about if we made the same uh sort of equation for the sheer |
|
| 117:41 | . So, so far as we sheer modules here and sheer modules of |
|
| 117:47 | uh I mineral here and sheer modules the I mineral here. Well, |
|
| 117:53 | is what we did um back Uh when we were talking about Voice |
|
| 117:58 | Hill averaging, but this sum includes porosity. So for the uh the |
|
| 118:04 | space, the uh the sheer modules the pore space is a zero. |
|
| 118:10 | you don't want to uh have zero the minus one power. That's a |
|
| 118:15 | thing to have. And so, uh this formula is uh uh one |
|
| 118:21 | uh we, we need to do than that we need. Uh This |
|
| 118:27 | OK. This one is false here's a question in the previous uh |
|
| 118:35 | previous question about the density. The one there also it says it includes |
|
| 118:41 | grains and the porosity. So the of the rock is for both, |
|
| 118:47 | just the minerals. That's correct. Yeah, so, so uh uh |
|
| 118:52 | for bringing that up. Uh uh we talked about this, we, |
|
| 118:55 | were talking uh uh we applied, discussed a similar expression only over the |
|
| 119:02 | . And so now we're including both and pores and we're just uh including |
|
| 119:07 | in the sum and, but we're just adding up the um the mass |
|
| 119:12 | the rock. So, so th one is valid even though the, |
|
| 119:15 | sum uh includes both grains and I uh it's a good point. |
|
| 119:20 | this is a good time to take break. So let's break for uh |
|
| 119:25 | 10 minutes and come back uh at uh at, at 1115 Houston time |
|
| 119:34 | we'll take up the issue of effective . No. So let's uh resume |
|
| 119:41 | this point. So affect your I think maybe you know what affect |
|
| 119:50 | pressure is. But I think, , yeah, you're probably wrong. |
|
| 119:54 | uh let's uh talk about this um . And first I wanna get myself |
|
| 120:02 | uh partner. Here's my partner. . Now, uh because the rock |
|
| 120:11 | not homogeneous, it's got greens and . So I, that means we |
|
| 120:16 | additional variables. We need to describe condition of the rock. For |
|
| 120:21 | the stress, we need to have average stress throughout the whole volume. |
|
| 120:26 | separately, we have to have the , the poor fluid pressure. This |
|
| 120:32 | an important idea. This was first uh uh given by uh a guy |
|
| 120:38 | bio in 1941. So at that , he was at Columbia University uh |
|
| 120:46 | as a professor there. But uh he made many important contributions to many |
|
| 120:54 | um areas of science, including for , aeronautics. Imagine it in |
|
| 121:02 | they have uh uh they know his and we know his name and Paul |
|
| 121:08 | . So the uh uh quite a guy. Uh So uh we need |
|
| 121:16 | specify in the rock at uh before wave gets there. And during the |
|
| 121:21 | of the wave, we're gonna have , a separate um uh stress conditions |
|
| 121:29 | of the homo, the heterogeneity of rock. So we have the average |
|
| 121:36 | and the fluid pressure. And uh , uh these uh might vary from |
|
| 121:43 | to place in the rock, think that. But uh uh for |
|
| 121:47 | all we're just saying is we need to specify the food pressure separately. |
|
| 121:53 | then the, the strain uh also gonna have a a so there's gonna |
|
| 121:58 | a, a separate strain in the uh uh in, in the pore |
|
| 122:04 | because of the heterogeneity because we have , we need to spec have these |
|
| 122:11 | variables to describe this. And the in the pore fluid is always gonna |
|
| 122:16 | a pure dilatation, pure volumetric contraction increase. And uh so I want |
|
| 122:24 | to be thinking about a closed system uh uh no fluid squirting out of |
|
| 122:29 | rock. Uh uh um only deforming as the wave goes through because of |
|
| 122:36 | fluid pressure is uh different than this stress. And so the average drain |
|
| 122:43 | gonna be different also in the two of the rock. Uh Now, |
|
| 122:47 | the laboratory, uh you can uh you can set up an experiment or |
|
| 122:54 | can independently adjust the pressure in the from the uh pressure in the |
|
| 123:01 | Anything you want, you just have little um uh membrane around the rock |
|
| 123:08 | a, a a AAA hole in membrane and a pipe coming out and |
|
| 123:14 | pipe can either inject fluid or withdraw , whatever the experimenter wants to |
|
| 123:22 | When we do things like that, observe that when we increase the average |
|
| 123:27 | that increases the density, I think quite uh uh quite obvious. Uh |
|
| 123:34 | when we uh when we uh uh the fluid pressure that decreases the |
|
| 123:42 | think about the rock as kind of a balloon. When you increase the |
|
| 123:48 | in a balloon, the balloon And so in the same way, |
|
| 123:55 | , the rock uh uh uh uh rock density decreases because of, of |
|
| 124:03 | fluid pressure increasing. So we have two effects. Uh uh And so |
|
| 124:14 | , what you could do is you change both the average pressure and the |
|
| 124:18 | pressure simultaneously in a certain uh uh . And so you could figure it |
|
| 124:25 | that uh uh for a certain uh of, of uh average pressure and |
|
| 124:31 | pressure, the density is constant. that combination, whatever it is, |
|
| 124:37 | of these two pressures, we call the effective pressure. Now, to |
|
| 124:44 | first approximation, the effective pressure is the differential pressure, simply the difference |
|
| 124:50 | the uh average pressure and the fluid . So to this approximation, if |
|
| 124:56 | increase the average pressure by a certain and you increase the fluid pressure by |
|
| 125:01 | same amount, the change in effective is zero. Uh uh more |
|
| 125:09 | uh uh the change in differential pressure zero. And so, since the |
|
| 125:16 | pressure is approximately equal to the effective , what that means is that in |
|
| 125:22 | operation that I just said the density unchanged? OK. Now, when |
|
| 125:29 | increase the, the average pressure that's make a uh a natural increase on |
|
| 125:35 | poor uh fluid pressure, but it's as much. So, in order |
|
| 125:41 | achieve an equal increase in flood you've got to uh uh inject some |
|
| 125:48 | into the rock sample. That's how do it. Otherwise, uh uh |
|
| 125:53 | condition of, of uh uh zero in differential pressure. That doesn't |
|
| 126:00 | Uh uh Because uh without the without the extra fluid, the uh |
|
| 126:08 | you squeeze it from the outside with A AAA given average pressure, the |
|
| 126:14 | pressure on the inside will increase but as much. So you gotta add |
|
| 126:20 | more fluid from the outside. When wave is traveling through a rock that |
|
| 126:25 | gonna happen. There's, there's no uh uh external reservoir for uh the |
|
| 126:31 | to come from. So when a travels through the rock, you never |
|
| 126:36 | , the effective pressure would be constant the wall. Now, you can |
|
| 126:41 | the same thing for uh uh uh velocities and for the stances, they |
|
| 126:48 | depend upon uh uh the average pressure the fluid pressure and only through a |
|
| 126:55 | , which is approximately given by this . So that means we can write |
|
| 127:06 | effective stress answer uh to be approximately to the, to the differential stress |
|
| 127:14 | . And we just add on the uh on the diagonal only on the |
|
| 127:19 | , we add the fluid pressure with minus sign because that's the convention and |
|
| 127:26 | off diagonal. Uh uh And uh are the same uh uh uh they |
|
| 127:33 | have the pressure in there. But is the average uh stress, the |
|
| 127:37 | 12 stress, this is the average stress. And since we're defining |
|
| 127:42 | the effective stress tensor, as it's by the differential stress tensor, we |
|
| 127:48 | a pore pressure here. The, poor fluid pressure has no off diagonal |
|
| 127:55 | right inside the, the fluid, uh uh the, the 12 component |
|
| 128:03 | fluid stress is zero because it's it's a, a fluid can only |
|
| 128:10 | a pure pressure in the fluid. so a another way to write this |
|
| 128:14 | thing is the average value of of the stress minus the fluid pressure |
|
| 128:21 | on the diagon. That's what this I this I vector has uh uh |
|
| 128:27 | only uh it has ones on the dagon and zeros off Dale when you |
|
| 128:37 | this, uh uh what you say that uh uh um uh be because |
|
| 128:46 | , we've done this for Tao and done it for um uh uh for |
|
| 128:52 | . We've done it for uh for longitudinal modulus M. And we've done |
|
| 129:00 | for sheer modernist move because of what we have uh did uh this |
|
| 129:06 | by this approximation is the P which in principle, differs separately on |
|
| 129:14 | two. Actually, it is gonna only on this difference and we're gonna |
|
| 129:20 | sloppy and we're gonna call that differential affecting pressure many times. And uh |
|
| 129:27 | we should be and in the back my mind that uh that actually it's |
|
| 129:32 | exactly the uh the effective pressure, not exactly the differential pressure. |
|
| 129:38 | to be more accurate, we could instead of uh uh the effective pressure |
|
| 129:44 | uh uh the differential pressure we could in here another constant and just give |
|
| 129:51 | name in a and that's gonna be empirical parameter. And when we do |
|
| 129:59 | , we find that N is not to one that is when we uh |
|
| 130:04 | uh vary the uh external pressure and fluid pressure independently, such as to |
|
| 130:11 | the uh the P velocity invariant, always find that N is not equal |
|
| 130:17 | one. And furthermore, it's not constant. It, it varies with |
|
| 130:22 | and, and uh fluid pressure. we should have here at the end |
|
| 130:28 | a FAA function of external pressure and pressure itself. So the combination is |
|
| 130:35 | uh uh this is in itself is and get this, it's gonna be |
|
| 130:43 | and it's gonna be different for the properties, right? Uh uh uh |
|
| 130:49 | velocity, sheer velocity, et So you see that uh that when |
|
| 130:53 | say that defensive pressure equals differential that's a real approximation. And we |
|
| 131:00 | to keep that in mind that it's common approximation, but keep it in |
|
| 131:05 | that that is uh um uh probably maybe an oversimplification. OK. But |
|
| 131:14 | , to pursue this line of uh analysis that it belongs in a different |
|
| 131:20 | , this is of course on wave . So we're going to stop with |
|
| 131:25 | discussion right here within the sub service zones where the poor pressure is anomalously |
|
| 131:34 | almost every time you drill um uh borehole into the earth in search of |
|
| 131:40 | . You find before you get to hydrocarbons, you find a layer or |
|
| 131:46 | several layers, several zones where the fluid pressure is anomalously high. We |
|
| 131:52 | these over pressure zones. And when driller uh uh drills into one of |
|
| 131:58 | , he immediately knows what's happening because the performance of his drill bit. |
|
| 132:04 | he can, I don't know imagine yourself as a driller. |
|
| 132:07 | what can you control there? Uh you're drilling, well, you can |
|
| 132:12 | the rate of rotation, you can the weight on the bit. You |
|
| 132:17 | control the weight of the, of density of the mud, which is |
|
| 132:21 | the hole and all. And uh so you have uh uh um these |
|
| 132:28 | are under your control as a driller that's determining the rate of penetration of |
|
| 132:35 | bore hole, you know, thousands feet down. Uh So drillers know |
|
| 132:39 | they're doing and when they encounter zones anomalously high pressure, uh uh they |
|
| 132:45 | what to do. And furthermore, know they, they know what |
|
| 132:49 | they better do it quickly because uh there could be a catastrophe. Uh |
|
| 132:55 | example, if they encounter a zone anomalously high pressure, uh that uh |
|
| 133:01 | pressure that might inject um, fluids , um I think the formation into |
|
| 133:09 | borehole, the borehole up the top uh uh uh spraying water all over |
|
| 133:16 | , uh, uh, uh, over the crew. Well, he |
|
| 133:19 | want that and, uh, worse can happen. Suppose he's drilling down |
|
| 133:24 | . He encounters, uh, an reservoir under high pressure and that oil |
|
| 133:30 | up and it, uh, uh, ignites when it gets |
|
| 133:34 | to the top and there's an explosion a blow out and everything. These |
|
| 133:37 | bad things. We don't want those to happen. So, uh, |
|
| 133:43 | usually what our our drillers say uh the geophysicists, they say tell us |
|
| 133:49 | your seismic data. You got all seismic data, we went out all |
|
| 133:53 | expensive surveying and all the expensive processing so on. Uh uh you're making |
|
| 134:00 | of the sub surface, tell us of these layers has high pressure. |
|
| 134:08 | um we do the best we We uh uh a company like slumber |
|
| 134:14 | will have experts who uh uh know to interpret the seismic data in terms |
|
| 134:21 | fluid pressures. But you will have that the uh the fluid pressure does |
|
| 134:27 | appear explicitly in any of the formulas we've talked about here. It's only |
|
| 134:35 | in those formulas. Now, we for example that in a a layer |
|
| 134:40 | anomalously high fluid pressure, the P velocity is gonna be anomalously low and |
|
| 134:48 | density is gonna be ply low and sheer wave velocity is gonna be a |
|
| 134:53 | low. We know those things in from years and years of laboratory |
|
| 134:59 | However, that low velocity could also from the fact that those rocks are |
|
| 135:06 | rock. Maybe we drilled into a coming out of a sandstone, into |
|
| 135:10 | shale would be a, a an where the decrease the observed uh uh |
|
| 135:24 | . That's uh uh you thinking of scenario where uh before we're drilling, |
|
| 135:31 | doing seismic acquisition processing and imaging and have a good image of the |
|
| 135:38 | And we also have an estimate of the velocity is like everywhere in the |
|
| 135:43 | . We see a zone where the is low. Now, before |
|
| 135:51 | we ask ourselves, is that due overpressure that throw down there of low |
|
| 135:55 | or is it due to soft For example, maybe that's a zone |
|
| 136:00 | shales or maybe it's a zone with of porosity, no normal pressure but |
|
| 136:06 | of pros or it might be a where the uh of course are shown |
|
| 136:11 | instead of with bright. All those are there. And because of this |
|
| 136:21 | , it's not easy to predict Before drilling, we often make |
|
| 136:29 | We do the best we can. advise our driller colleagues. Uh |
|
| 136:32 | Down there is a, a zone low velocity, we think that's due |
|
| 136:40 | overpressure and how much overpressure is it to, we can tell them that |
|
| 136:47 | usually rock. We, we're wrong the depths to the uh to the |
|
| 136:53 | and we're wrong about the amount of . But at least we have, |
|
| 136:58 | , we've uh given a heads up the driller. So when he gets |
|
| 137:03 | there, he's paying close attention to drill bit performance. And he sees |
|
| 137:10 | the, um, the rate of suddenly begin gets to be higher. |
|
| 137:18 | he knows then that, uh, , uh, that's really an anomalous |
|
| 137:22 | . He just, uh drilled it could well be due to |
|
| 137:26 | So, the first thing he does he increases the weight of the mud |
|
| 137:32 | the, um, borehole. And , you know, he does that |
|
| 137:35 | the top. And so that increases , uh, uh, the weight |
|
| 137:39 | the, uh, of the uh, down at the bottom of |
|
| 137:44 | war hall. And that, uh, keeps the high pressure from |
|
| 137:49 | surrounding rocks from coming into the And he proceeds cautiously. He doesn't |
|
| 137:56 | that. There, there could be blowout and I'm sure you've seen all |
|
| 137:59 | pictures of, of, uh, , of blowouts and, uh, |
|
| 138:04 | early days of drilling where they didn't really what they were doing. And |
|
| 138:08 | modern cases, you, you know the case of, uh, the |
|
| 138:12 | in, uh, the Gulf of , uh, in TW, in |
|
| 138:16 | year 2010. So you were all younger than them, but I'm sure |
|
| 138:21 | know about that blow up blowout. , it's a block out that I'm |
|
| 138:28 | familiar with because it was a BP , well, it blew out. |
|
| 138:35 | called it an oil spill but it really a blowout and it happened after |
|
| 138:40 | retired from BP. So it was my fault. Uh, uh, |
|
| 138:45 | you've all seen those pictures and you've the stories and, uh, that |
|
| 138:50 | out was a very serious event in history of BP. It almost destroyed |
|
| 138:56 | company. Uh, the company has , uh, up to this point |
|
| 139:01 | $60 billion but they weren't planning to because of that blow up billion with |
|
| 139:09 | B that's a lot of money even a Greek companies. So, um |
|
| 139:14 | we had BP experts uh uh on the job, of course, |
|
| 139:19 | they did not adequately uh predict uh pressure and it's, it only partly |
|
| 139:28 | fault. Uh uh um uh because the ambiguity that I'm mentioning here, |
|
| 139:34 | can't do that as geophysicists. We do that. Um uh Precisely. |
|
| 139:40 | it's a bit unfair to say that blowout at, at the VP. |
|
| 139:44 | , in 2010, that's called the Blowout because we had given the name |
|
| 139:49 | the reservoir macondo uh uh before the started. Uh So that that event |
|
| 139:56 | only partly the fault of uh the uh who were working for BP and |
|
| 140:04 | the contractors at that time. So of that uh was a traumatic |
|
| 140:13 | but uh it sort of lies outside scope of this course. So we're |
|
| 140:19 | gonna talk about those sorts of events . But I do have a quiz |
|
| 140:25 | here and I'm gonna start with the . Uh Which of the following statements |
|
| 140:32 | the, is the best way to the following statement. The mechanical properties |
|
| 140:37 | rocks, including velocities are determined by or D. So let me, |
|
| 140:43 | , uh start with a, uh . Would you say that a proper |
|
| 140:48 | to complete the sentence is to add the phrase or pressure? Um |
|
| 140:55 | it's, it's, it's, it's but it's not the be, it |
|
| 140:58 | not be the best. But so, so we can, and |
|
| 141:01 | need to go down and look at best. So uh uh over to |
|
| 141:05 | Lili, how about uh is overburden stress? Uh uh the best way |
|
| 141:10 | complete the sentence? He says, , he said it very softly but |
|
| 141:14 | can hear it in here. uh over to you, Carlos uh |
|
| 141:18 | it the difference between overburden pressure and pressure? Is that the way the |
|
| 141:24 | way to uh complete this sentence Carlos? I wanna hear you thinking |
|
| 141:34 | loud. Yeah, I, I that one is true professor. |
|
| 141:41 | that one, that one, that is uh uh defective pressure, uh |
|
| 141:46 | effective pressure. That, that's down . Indeed. That uh so |
|
| 141:50 | I'm gonna agree with you. It's effective uh stress which is uh um |
|
| 141:54 | the best way to complete it. we are commonly sloppy and we commonly |
|
| 141:59 | the difference. But uh let's recognize that is uh uh uh that's an |
|
| 142:06 | . And it's really this combination of we call the effective stress. That's |
|
| 142:11 | best way to complete this statement. . So with this understanding that |
|
| 142:17 | we now have the beginnings of an of coral elasticity. So now let's |
|
| 142:22 | apply that to body works. So our principal aim is to understand the |
|
| 142:30 | of fluids on sizing velocities. This obviously gonna be important for amplitude analysis |
|
| 142:36 | we did in lecture six that so we understand this properly, we will |
|
| 142:42 | a direct detector of subsurface fluids, uh de detector in the s data |
|
| 142:50 | the subsurface fluids. And uh uh uh maybe we can do that. |
|
| 142:56 | We talked about it in electric We're gonna talk about it more just |
|
| 143:00 | and we're gonna talk about that topic uh on uh on the next two |
|
| 143:07 | also. So this business, the of fluids on the seismic velocities is |
|
| 143:14 | be important for four D interpretation because we uh make a seismic image before |
|
| 143:22 | and then we uh drill and produce make another se image the fluids have |
|
| 143:27 | inside that uh reservoir. And we see the changes uh in from the |
|
| 143:34 | and the differences in the seismic data in the reshoot. Uh Even though |
|
| 143:42 | uh uh even though the reservoir is thousands of feet down. It makes |
|
| 143:48 | on seismic data, which we can . And that's crucial for our understanding |
|
| 143:54 | how to uh uh to reduce the , uh produce oil from that bore |
|
| 143:59 | . And also, you know, , where to place the next |
|
| 144:03 | Usually, uh it's not sufficient to only one bar, usually most reservoirs |
|
| 144:08 | we are big enough for us to into, you know, because it's |
|
| 144:12 | cost us millions and millions of dollars drill into that. Uh Usually |
|
| 144:17 | it's uh to justify that drilling it better be a big reservoir and |
|
| 144:23 | it means more than one borehole or might be a borehole which is uh |
|
| 144:29 | horizontally. So it stretches away from wellhead to a distant portion of the |
|
| 144:36 | . All of these are things that re are engineer friends are uh doing |
|
| 144:42 | on our four D interpretation of cycling . So we're gonna uh analyze the |
|
| 144:47 | and the elastic moduli separately. So , let's assume uniform grains and recognize |
|
| 144:54 | the pressure in the fluid is different the pressure in the solid. And |
|
| 145:00 | the strain in the fluid is different the strain in the solid. And |
|
| 145:04 | uh is uh true uh before the gets there while it's uh it's uh |
|
| 145:10 | the effect of it of large initial and large initial core pressure. And |
|
| 145:17 | when the wave gets there, it's uh um affect the uh the, |
|
| 145:22 | , the small additional wave pressures inside wave are gonna be different in the |
|
| 145:29 | in uh different in the fluid than the solid. And also the strain |
|
| 145:33 | gonna be different in the fluid than solid. So, let's consider |
|
| 145:39 | a mass element. We can call a max. Uh uh it, |
|
| 145:44 | like a pixel except a pixel is two D element of a, of |
|
| 145:49 | two D image. So we're gonna about a 3d element with, with |
|
| 145:54 | fixed mass. We don't call it voxel. A voxel is an element |
|
| 145:59 | volume, but sometimes that's, you , deceptive. And we're gonna call |
|
| 146:06 | a max to emphasize the mass inside element is unchanged throughout the passage of |
|
| 146:15 | way. If we had a voxel the laboratory where it's connected to an |
|
| 146:23 | reservoir, that Vaux salt might not constant mass as the wave goes |
|
| 146:31 | It might be uh squeezing water in and out of the external reservoir in |
|
| 146:38 | laboratory. So that can't happen in uh in the rock because there's no |
|
| 146:44 | laboratory. So let to emphasize that , we're gonna call it a |
|
| 146:50 | It's gotta be large enough to contain in many grains are small enough. |
|
| 146:54 | the average stress and strain is not much across that volume. So uh |
|
| 146:59 | gonna be smaller, a lot smaller a seismic wavelength, for example. |
|
| 147:07 | we're gonna uh uh uh think about situation and we're gonna conclude that the |
|
| 147:12 | that within the uh heterogeneous rock sample to point variation within the solid is |
|
| 147:19 | less than the difference between solid and . So, uh because the solid |
|
| 147:25 | heterogeneous in itself, it has um many different minerals. Uh uh |
|
| 147:31 | is gonna be a, a point point variation of threatened strain inside the |
|
| 147:37 | , but we're gonna ignore that and , the main thing we have to |
|
| 147:41 | is the difference between the stress and in the solid and the stress and |
|
| 147:46 | in the rock. Furthermore, we're assume that the poor fluid pressure is |
|
| 147:54 | within the maxim. So this implies frequency. If we did high |
|
| 147:59 | then as that high frequency wave was through the rock, uh uh it's |
|
| 148:05 | be a, a moving fluid from parts of the rock to other parts |
|
| 148:09 | the rock. We call that we call that fluid squirt inside the |
|
| 148:13 | at the grand scale. Uh uh we're gonna do this at low |
|
| 148:19 | And so we're further gonna assume that band uh uh excuse me, seismic |
|
| 148:25 | frequency is low enough. Yeah. uh People generally agree that is low |
|
| 148:32 | but uh we know ultrasonically that's not enough and sonic may or may not |
|
| 148:38 | low enough uh depending on um uh litho we're talking about, but everybody |
|
| 148:44 | that we can usually use this assumption uniform po po pressure in a seismic |
|
| 148:52 | . OK. Now, under these , Leo proved in 1941 that for |
|
| 149:01 | uh ordinary sound waves, we have velocities, a VP and A vs |
|
| 149:06 | , of course. And they depend uh the uh uh something we're gonna |
|
| 149:13 | the uh the undrained density and the in un in compressibility and the undrained |
|
| 149:22 | modules. So we uh we uh long as we have no fluid leaving |
|
| 149:31 | sample as the wave goes to uh straightforwardly use Coke's theory, you |
|
| 149:42 | , that's really good. So this undrained means that no fluid enters or |
|
| 149:53 | the max. So we can use and all we have to do is |
|
| 149:59 | that these parameters depend upon the composition of the uh solids and the composition |
|
| 150:07 | the fluids and the fluid pressure and , the external pressure. All of |
|
| 150:13 | uh uh uh as I all these complexity is implicit inside here. So |
|
| 150:19 | looks like a straightforward generalization of But remember that implicit inside this is |
|
| 150:28 | on all these things. And and we, we need to understand |
|
| 150:33 | , but this is really good We can use everything that we talked |
|
| 150:37 | in the first seven lectures if the are isotropic, even though they're not |
|
| 150:44 | because they all proved that uh not , a trivial um not, not |
|
| 150:51 | trivial. They are amazing. But agree starting in 1941. And with |
|
| 150:58 | advances since then, we can get with applying a hook flaw into non |
|
| 151:07 | rocks. If we just recognize, got to insist that the fluids don't |
|
| 151:12 | out. And uh uh we recognize we're gonna have a dependence of all |
|
| 151:17 | things on the, the composition of grains and the composition of the |
|
| 151:25 | the pressure on the grains and the pressure. Awesome. Now, under |
|
| 151:32 | conditions, under these assumptions, the is easy to analyze because we're just |
|
| 151:38 | up. Uh Professor just a, comment. I, I think the |
|
| 151:43 | light is not in the, in notes that you shared with us. |
|
| 151:53 | . So uh uh uh thanks for . I will uh look at |
|
| 151:58 | It might be that um the file I uploaded to you had that slide |
|
| 152:04 | be hidden, but I hope you this slide and is the one it |
|
| 152:10 | I will uh provide to you um um or maybe even over lunch, |
|
| 152:16 | provide to you uh overnight tonight. in this. Thank you. And |
|
| 152:25 | you know, um you might have other slides in the past few weeks |
|
| 152:34 | what I'm showing you is not precisely is in the file. That's because |
|
| 152:38 | made some small changes, improvements uh I uploaded the files to canvas and |
|
| 152:45 | didn't think that they were. So so um uh important changes that I |
|
| 152:51 | to change canvas as well. But one is important. So I'll go |
|
| 152:55 | and make sure that that slide is the file that you have now. |
|
| 153:04 | So uh this is an easy extension uh the ideas we had about uh |
|
| 153:10 | dens, the average density in the . Here, we are including the |
|
| 153:14 | . And uh here we're just adding all the uh the um the density |
|
| 153:21 | uh in the solid. And of course, in the uh uh |
|
| 153:26 | the, the density in the solid also gonna uh dependent, be dependent |
|
| 153:33 | the composition of the solid. So we have the saturation fraction of |
|
| 153:40 | saturation fraction of oil and sat saturation of gas all together inside here. |
|
| 153:47 | of course, these saturation f factors to add up to one. So |
|
| 153:52 | think that's all uh uh uh pretty . And uh here's the, the |
|
| 153:57 | um notation for this. Uh So was easy for the density complicated, |
|
| 154:05 | would say, but, but And so I, I now return |
|
| 154:09 | the stiffness. So, and uh commonly understands these because of a contribution |
|
| 154:15 | Gasman in 1951. And this is years after bio did his work, |
|
| 154:22 | uh did his work in 1941 and did this in 1951. And of |
|
| 154:31 | , in the uh in the we had a war. And so |
|
| 154:38 | Gasman is German and Biau is Belgian be French, but he Belgian. |
|
| 154:46 | you can imagine these two guys, and Gasman were occupied with other uh |
|
| 154:53 | issues during the war. And uh the war, Gasman published his theory |
|
| 155:00 | the effects of fluid content and shame him, he did not mention via |
|
| 155:07 | should have. So here's what uh and by the way, um uh |
|
| 155:14 | uh we understand this uh theory is to gas mon, but it was |
|
| 155:21 | , it was actually this part of was actually uh originated by Brielle 10 |
|
| 155:28 | earlier and Gasman did not recognize So, what both these guys agree |
|
| 155:36 | is that uh the sheer margins is by the presence of the fluid. |
|
| 155:44 | , if you have uh uh the uh the shear modulus for an undrained |
|
| 155:49 | , that's exactly the same as the fluid modulus for uh uh the same |
|
| 155:56 | with all of the oh uh all the fluid drained out. The, |
|
| 156:03 | sheer stiffness depends only on the framework the grains. So we didn't call |
|
| 156:09 | thing to be the drained shear We call it the frame shear models |
|
| 156:15 | uh that is uh directly uh the miners of the, of the empty |
|
| 156:22 | frame alone. So everybody agrees on and then uh uh uh the next |
|
| 156:31 | . So just professor, just to . So it doesn't matter if it |
|
| 156:35 | a fluid or not, the shared will be the same. That's correct |
|
| 156:40 | you can think about that as uh you shear a rock, the fluid |
|
| 156:44 | gonna shear without resistance because uh uh , the fluid has zero shear modulus |
|
| 156:52 | the fluid. Uh uh uh uh , so when you shear the |
|
| 156:56 | it doesn't, the, the flute of the rock does not resist the |
|
| 157:01 | that you put on the outside. it only depends on the, |
|
| 157:09 | your stiffness of the framework of the . Now, that's not the same |
|
| 157:16 | the sheer stiffness of the solid grains uh the solid grains are assembled into |
|
| 157:23 | framework with a complicated uh horse space between. So uh the, the |
|
| 157:34 | modulus of the frame is different from sheer mos of the solid. But |
|
| 157:40 | , you, you can uh you measure it if you want, you |
|
| 157:43 | measure it uh by simply by going the laboratory allowing all the fluid to |
|
| 157:48 | out and share it um without anything the pore space. And you'll find |
|
| 157:55 | the same as, as if you fluid in there. Yeah. But |
|
| 158:04 | this frame here is the same as uh as Andre two different ways of |
|
| 158:12 | the same thing. Now, for in compressibility, it's more complicated. |
|
| 158:18 | the formula and here's all the So let's look at this. It |
|
| 158:23 | that the uh the incompressible of the rock differs from the in compressibility of |
|
| 158:32 | empty frame by this expression here. what's in this expression? Well, |
|
| 158:38 | have the in the in compressibility of fluid see that uppercase F is the |
|
| 158:44 | or is the lowercase fr is the . So this is the incompressible, |
|
| 158:50 | the food, you know, it's be different for uh oil or for |
|
| 158:54 | or for gas. And then also see explicitly the uh uh the in |
|
| 159:01 | of the solid grains. And here have explicitly that uh the incompressible of |
|
| 159:07 | framework, this number is the same this one, but it's different from |
|
| 159:11 | one. And uh so that means since these two are different, that |
|
| 159:17 | that the uh you know, this not a zero. If, if |
|
| 159:21 | frame, if the incompressible compressibility of frame were the same as the incompressible |
|
| 159:27 | the solid, then making this ratio be a one and this would be |
|
| 159:31 | zero. But that doesn't happen. course, the framework has a as |
|
| 159:36 | smaller in compressibility in the solid But this ratio is a number less |
|
| 159:42 | what and it gets subtracted off of and squared. And it makes uh |
|
| 159:49 | uh shows the difference between the compressibility the frame in compressed melty. Now |
|
| 159:58 | here that we are not uh the uh gas man and we |
|
| 160:03 | nobody will tell you what this framework . This is incompressible to the frame |
|
| 160:10 | uh it only gives you the fluid and the fluid dependence involves that, |
|
| 160:15 | know, in compressed building. So , if I tell you, if |
|
| 160:19 | , if I, if we have rock sample and we look at it |
|
| 160:22 | holding it in our hands and we say, see, for example, |
|
| 160:26 | it's a piece of sandstone and it's 80% quartz crystals, quartz grains and |
|
| 160:34 | . Um uh Glad you collect, can uh probably maybe see that with |
|
| 160:41 | eyeball or maybe with a little an optical microscope and uh our p |
|
| 160:47 | friend, uh uh we can see and also we can see that the |
|
| 160:55 | process say is 25%. So we see all that with a Mac with |
|
| 161:01 | simple observation of the rock sample So we can't say what is the |
|
| 161:08 | compressibility of the frame? Because the space is so complicated. We can't |
|
| 161:17 | you that uh the sheer models of frame or the in compressibility of the |
|
| 161:23 | . Neither one of these numbers, can't tell you even though we have |
|
| 161:27 | rock in our hand and we can all kinds of stuff in the |
|
| 161:30 | We can't tell you that without directly . So if, if you wanna |
|
| 161:35 | what is the incompressible of the you've gotta actually measure it in the |
|
| 161:41 | . And the reason for that is um that uh the complicated shape of |
|
| 161:49 | pore space in there and also also complexity due to the mineral heterogeneity. |
|
| 162:02 | , let's talk about that. Just little bit more. Suppose that uh |
|
| 162:07 | uh let me change the model. we have a sandstone but it's got |
|
| 162:12 | of clay in there. It's got , it's got some clay grains in |
|
| 162:15 | . Not, not to call it shale. Let's say, let's |
|
| 162:18 | uh uh 20% clay and 80% quartz the minerals. But you know, |
|
| 162:27 | clay is gonna be a lot softer the quartz. So I imagine that |
|
| 162:34 | um this rock has the soft play between the um uh the the stiff |
|
| 162:46 | grains. So that's one possibility. one, another possibility is that you |
|
| 162:52 | quartz. Uh yeah, uh quartz contacted directly with another quartz crystal and |
|
| 163:05 | the clay is off to the It's lining the inside of the quart |
|
| 163:11 | . In that case, the stiffness the rock is gonna be uh it's |
|
| 163:15 | be stiffer because we have quartz on and the dependent and the softness of |
|
| 163:20 | clay is not so important. This a good visual example of how well |
|
| 163:31 | uh the frame compressibility depends not only the composition but also on the microscopic |
|
| 163:39 | of the different minerals inside the I think you have a good picture |
|
| 163:45 | that. Now. Uh uh uh that is why even uh that's why |
|
| 163:55 | we did not derive the properties of rock uh uh as a uh or |
|
| 164:04 | didn't drive even the, the pro of the uh of the grains. |
|
| 164:14 | uh we could only uh uh drive upper bounds and lower bounds and it |
|
| 164:19 | be somewhere in between. The basic why we couldn't do any better than |
|
| 164:24 | is because the incompressible if of a like that depends upon the details of |
|
| 164:30 | micro geometry. And usually you don't much about that. You can look |
|
| 164:36 | it on, on the microscope and can see that for this part that |
|
| 164:39 | looking at this is the the uh , but it's hard to make a |
|
| 164:46 | uh with consonants, any statement about microscopic arrangement of the heterogeneity in a |
|
| 164:56 | . And that's true, even when concentrating only on the solid part of |
|
| 165:00 | solid, you, you got Uh uh you know, intuitively, |
|
| 165:06 | know that the the stiffness of the depends upon the microscopic geometry of the |
|
| 165:14 | um mental constituents. But you don't what that that is. So the |
|
| 165:21 | way you can uh find out about frame of the, of the in |
|
| 165:27 | and the shearers of the frame is in the laboratory and do experiments. |
|
| 165:36 | , what gas mines, this is uh this is the result specifically due |
|
| 165:41 | gas mine 1951 Gasman is telling you what is the fluid dependence. So |
|
| 165:50 | the end rock, uh uh you have a loose air in the force |
|
| 165:55 | . So this is, this is uh a zero for the incompressible of |
|
| 166:00 | air and that uh po space. so that, that makes the, |
|
| 166:05 | uh the whole side here is uh zero. So we have zero to |
|
| 166:11 | minorly power that's infinite and infinite in um in the denominator that makes the |
|
| 166:16 | thing to be zero. So uh when you uh this, this |
|
| 166:25 | what this expression shows you when you some fluid with the uh with a |
|
| 166:31 | uh fluid in compressibility that is going be increasing this grain um stiffness, |
|
| 166:41 | it's not gonna be changing this one this one is, is due only |
|
| 166:45 | the Frank. So what Gasman predicted the fluid dependence, nothing more. |
|
| 166:55 | he published it in German in 1951 this says on the elasticity of pus |
|
| 167:02 | , you can sort of see but most of us don't speak German |
|
| 167:06 | very well. Uh And so most us, I didn't read this uh |
|
| 167:14 | carefully. In 1951 we use the , but we didn't um uh we |
|
| 167:23 | really read the paper carefully because we speak German. Well, this was |
|
| 167:30 | problem. And in the year the SCG translated this got a bunch |
|
| 167:37 | uh smart Jesus together and translated And so uh you can find this |
|
| 167:43 | uh published by the SEG in uh uh 1951. And, oh |
|
| 167:50 | here are the guys, here are people who translated it. And uh |
|
| 167:55 | , um if you're interested, if don't have this in, in English |
|
| 168:00 | if you're interested in getting in, English, um um write to me |
|
| 168:05 | I will give you a reference uh to, to the exact uh a |
|
| 168:13 | . Uh Matter of fact, I will send you a preprint of |
|
| 168:16 | paper, uh not a preprint, I'll send you, I'll send you |
|
| 168:19 | PDF file of uh this paper in . Now, everybody has to believe |
|
| 168:26 | . Uh starting in 1951 we believed . And after it was published in |
|
| 168:32 | uh after it was translated in we, we believed it got, |
|
| 168:40 | know what it's not really true. let's uh uh look at some um |
|
| 168:51 | support. So this is work that did a long time ago, maybe |
|
| 168:57 | some of you all we were born I, and I was working for |
|
| 169:01 | in the Rock Physics Laboratory. We measuring the VPN vs on lots of |
|
| 169:06 | and this is all. And I didn't do any of that. |
|
| 169:09 | that was uh done by my But um uh I made this graph |
|
| 169:20 | their data. And so this is we observed and this is what we |
|
| 169:23 | . Uh uh uh from the from gas mot. And he predicts they |
|
| 169:29 | be the same. So, so line is at 45 degrees and the |
|
| 169:33 | generally cluster along that line with some . But uh uh never mind the |
|
| 169:39 | , we can say that with uh some scatter. Um uh it's generally |
|
| 169:45 | uh Gas Mon's prediction, the first of that, this part here. |
|
| 169:52 | now for the other part, not see that the other part shows a |
|
| 169:58 | of deviation and always the observe is , too high compared to the |
|
| 170:05 | So uh so this, this uh is what? And hm I think |
|
| 170:21 | uh oh yeah. So this arrow pointing at a spot which you can't |
|
| 170:27 | because of the arrow and it was over here at a lower value. |
|
| 170:32 | there are lots of cases. Most the cases are the, the prediction |
|
| 170:38 | too low and it is too uh uh to the left of, of |
|
| 170:44 | was observed. So let's look at single one of those as a function |
|
| 170:49 | pressure. This is at uh uh uh Bria sandstone. Famous uh lots |
|
| 170:55 | experimentalists work on this sandstone comes from quarry in the American State of |
|
| 171:03 | And this is data acquired by Arthur Cheng. Do you know that |
|
| 171:08 | Arthur Chen? You should know he's president of the SCG and he did |
|
| 171:16 | a long time ago when he was know, uh uh he was uh |
|
| 171:19 | worker in the laboratory but uh here's Chang, he's uh the president of |
|
| 171:24 | SCG. By the way, he the first Chinese president of the |
|
| 171:29 | But I can guarantee you he will be the last, might be somebody |
|
| 171:35 | , that, you know, uh uh le le maybe somebody, you |
|
| 171:39 | , Utah, maybe somebody here at University of Houston. Uh uh uh |
|
| 171:46 | so this was done by uh Chen when he was at the National University |
|
| 171:51 | Singapore. Uh Excuse me, he this when he was at mit as |
|
| 171:58 | of Technology in America. But then uh I subsequently moved to the Singapore |
|
| 172:05 | at that time, he gave it me. And so this data is |
|
| 172:09 | . So here we have as a of confining pressure. Uh um uh |
|
| 172:16 | uh oh OK, the data that that Arthur acquired is flying along here |
|
| 172:25 | uh you know, it's decreasing as function of pressure just like we uh |
|
| 172:33 | oh excuse me, what we said that the, the, the incompressible |
|
| 172:39 | going to increase as a function of . What we're splitting here is the |
|
| 172:45 | between saturated and uh and framework. that difference is decreasing as a function |
|
| 172:52 | pressure. And the gas mo theory also decreasing. But it, |
|
| 172:56 | it's everywhere, it's lower. So discrepancy and furthermore, you can see |
|
| 173:03 | discrepancy is pretty big here and less here. So the discrepancy decreases as |
|
| 173:09 | pressure increases. And what's this due it's due to the closing of |
|
| 173:14 | So, as uh the pressure is cracks got closed. And so uh |
|
| 173:22 | the gas man theory got better and , but, you know, ga |
|
| 173:28 | line should have been uh um ex red curve should have been on top |
|
| 173:32 | the blue curve everywhere. Gasman made uh uh ee everywhere shows that Gasman |
|
| 173:39 | wrong and just the fact that he's as wrong at high pressure as |
|
| 173:44 | Uh That's not uh an issue. made no explicit assumptions concerning the poor |
|
| 173:52 | GME. Let's back up here. , you see there's no nothing in |
|
| 173:58 | about the poor micro geometry except as appears implicitly inside here inside the, |
|
| 174:07 | uh incompressible of the frame implicitly is effect of whatever cracks are there. |
|
| 174:15 | don't affect the solid itself and the don't affect the fluid itself. But |
|
| 174:19 | an implicit uh uh dependence on crack inside here. But uh going forward |
|
| 174:28 | the data, Gasman got it wrong , at all cases. So we've |
|
| 174:37 | this for a long, long time gas mon should not be applied to |
|
| 174:42 | to, to this kind of But we've ignored that anyway and used |
|
| 174:48 | anyway because we know that these ultrasonic that uh Chen did in the laboratory |
|
| 174:55 | high frequency and we know that the line theory is applicable only at low |
|
| 175:01 | . So we have ignored these uh discrepancies all these years for a |
|
| 175:08 | long time. Um So shame on that. Um, and that we |
|
| 175:25 | that only out of faith, why we do that? Didn't have any |
|
| 175:31 | um justification for that. So we assumed that the gas monitor theory is |
|
| 175:40 | and we assumed that on faith what should we have done? What |
|
| 175:44 | should have done was go back into laboratory and do those kinds of tests |
|
| 175:50 | the laboratory at low frequency. But a difficult experiment. Only a few |
|
| 175:56 | around the world can do that. mostly they haven't done. So mostly |
|
| 176:01 | we would say that the, the gas line theory has very low |
|
| 176:07 | support. No. Um Of we've measured ultrasonic velocities on lots of |
|
| 176:16 | . So how do we use that uh understand sing data? Well, |
|
| 176:21 | what we do is we measure we do the ultrasonic velocities on dry |
|
| 176:27 | in the laboratory. Why? It's they have no uh they don't have |
|
| 176:32 | effect of the fluid. Uh There no fluid squirting around inside the pore |
|
| 176:39 | of um uh those rocks. And we compute the saturated velocities using gas |
|
| 176:47 | . And uh uh uh Furthermore, uh well, that's what we |
|
| 176:53 | Almost everybody does that. I'm gonna you why that's not valid because of |
|
| 176:59 | arguments which follow. OK. Now assumed that the solid is micro |
|
| 177:06 | that is only one meal. We that's not true, but we're gonna |
|
| 177:12 | that uh uh difference because uh both are more similar to themselves than they |
|
| 177:17 | to Brian. And so this issue always been recognized and always deemed to |
|
| 177:22 | a minor issue. Here is something people never talk about. Since the |
|
| 177:29 | are anisotropic, they've got to be oriented. So the rock itself is |
|
| 177:35 | . When they, when you randomly them, there's gonna be places inside |
|
| 177:40 | rock where stiff axes of some crystals juxtaposed against soft faces in other |
|
| 177:48 | but not in other places. So can call this orientation in a homogenic |
|
| 177:55 | in homogeneity of anisotropic crystals that's also ignored without even talking about without, |
|
| 178:03 | even recognizing that's a problem. So gonna accept however, that that's that |
|
| 178:10 | of these issues are minor issues. . Now we're gonna rewrite gas man's |
|
| 178:18 | in this way only uh in this the following analysis, it'll be easier |
|
| 178:24 | us to talk about compressibility K Kappa of in compressibility K. So the |
|
| 178:33 | are simply the inverses of the And so then when you rewrite this |
|
| 178:38 | in terms of Kappas, uh uh see uh it looks the, the |
|
| 178:43 | term looks the same uh very but uh maybe even simpler, but |
|
| 178:49 | got a minus sign here because we're about the inverses of the cave. |
|
| 178:56 | this uh the, the compressibility of undrained rock uh is going to be |
|
| 179:03 | uh less than the compressible to the trimmer. It'll be stiffer. That |
|
| 179:09 | it's gonna be less compressible. So need this minus sign here. |
|
| 179:14 | all of this work, this is Monsters out here. But I told |
|
| 179:19 | before, poor elasticity elasticity was actually much earlier by Beau. There's a |
|
| 179:26 | of Bee, I think I have picture of Gas Mart. And so |
|
| 179:30 | is his paper he received as submitted the journal in 1940 but was not |
|
| 179:36 | by Gas Mont shame on him. it was B os innova innovation which |
|
| 179:42 | said that the Cheer models uh does depend on flood content. So we |
|
| 179:50 | agree on that. Uh um at frequency, we all agree on |
|
| 179:57 | Now, this is what the OS was. His essential contribution was. |
|
| 180:03 | said that for elastic, for homogeneous , we're gonna have classical elasticity and |
|
| 180:08 | gonna express Hook's law in this, this way here. Uh If we |
|
| 180:15 | an in homogeneous body, uh uh yeah, classical electricity is gonna say |
|
| 180:23 | , we just take these uh we it up. So the average dilatation |
|
| 180:28 | gonna depend upon the average in compressibility this way. And uh in terms |
|
| 180:34 | Kass this way, no big deal you have in a homogeneous bodies. |
|
| 180:40 | if there's porosity involved with fluids, bo said we need to do |
|
| 180:46 | We need to add another term. that the, the rotation is gonna |
|
| 180:52 | dependent on the compressibility of, of empty frame with an additional term with |
|
| 180:59 | additional ad parameter in it, which called H you might not be too |
|
| 181:07 | with this formula, but this is more or less directly from bio in |
|
| 181:17 | . Now, he, his focus on consolidation with expelling the fluid. |
|
| 181:25 | that's not what, what we We want uh a wave propagation where |
|
| 181:30 | food is uh uh undrained. but as end points of the, |
|
| 181:37 | this consolidation process, he defined the compressibility at the instant of application of |
|
| 181:44 | load. And we're gonna call that in compression ro now that we |
|
| 181:54 | we have to recognize it uh or have to, when he says instantaneous |
|
| 182:00 | , he doesn't mean exactly instantaneous. means that after all the local in |
|
| 182:06 | of uh in the poor fluid pressure been equalized out, that's the undrained |
|
| 182:13 | belt. And then finally, where uh uh fluid has all been squeezed |
|
| 182:18 | , the final compressibility is supported only the framework. And he doesn't mean |
|
| 182:25 | cases where the um the compressibility is in compressibility, uh you know, |
|
| 182:35 | out the pores, the pork space is not being squeezed out only the |
|
| 182:49 | . So, in this in this , the instantaneous dilatation is proportional to |
|
| 182:54 | under in compressibility. With this additional here introduced by be. And now |
|
| 183:03 | have to consider uh separately what's going in the poor space. So this |
|
| 183:08 | , this is the average for the . But inside the poor space, |
|
| 183:13 | wrote a similar expression. Uh This for the, the flu vol volume |
|
| 183:21 | and he introduced a second or elastic or elastic parameter R. And also |
|
| 183:29 | see in there the formula, the H one. Well, he showed |
|
| 183:33 | H one is uh uh equal to . So we don't have to worry |
|
| 183:39 | that. Now, if the rock drained, so nothing is actually draining |
|
| 183:44 | . Then the specific fluid content that showed on the previous slide, that's |
|
| 183:48 | same as the port fluid change. uh uh so here's the change in |
|
| 183:55 | , that's the change in the ferocity uh in the, in the poor |
|
| 184:02 | . It's given by the fluid in and the fluid pressure and the same |
|
| 184:09 | on the right. And so if eliminate the ratio of external pressure to |
|
| 184:15 | pressure, we find immediately this expression the difference between the undrained compressibility and |
|
| 184:21 | frame compressible. It, it uh took only uh uh two steps and |
|
| 184:28 | that we have these two new parameters by BO and he didn't tell us |
|
| 184:35 | they mean. He just showed them he said, OK, these are |
|
| 184:38 | parameters that we need for the He didn't talk about them a |
|
| 184:44 | Furthermore, he didn't talk about 3D compression. He only talked about one |
|
| 184:50 | compression. So I'm gonna say that's uh uh uh that, what we |
|
| 184:56 | here is the 3D expression of um formula in bio's 1941 paper. And |
|
| 185:05 | formula only applied to one D, this is generalizing from one D to |
|
| 185:11 | , it's AAA minor point. And I'm gonna uh call this uh bios |
|
| 185:17 | , not my bios expression. So I want you to uh compare these |
|
| 185:22 | expressions for um from gas mod and bio. And you see, |
|
| 185:28 | they have a lot of similarities, they? Uh the correspondence to notation |
|
| 185:34 | that the inverse of H is given this difference, you see it's squared |
|
| 185:39 | this is what's squared up here and had the same concept except he called |
|
| 185:45 | . Um H and down here, can see that uh the R in |
|
| 185:51 | is uh uh related to, to one expression by this summer term. |
|
| 186:05 | it also involves the uh uh the primer same as we had up |
|
| 186:10 | But what, what's new is it's the, the, the inverse of |
|
| 186:15 | S. So gam uh Gas Mon has replaced two GEOS two primer H |
|
| 186:22 | R with one para which is Kappa . And so that was done by |
|
| 186:30 | Mont in 1940 51 did not mention bill. And basically, nobody paid |
|
| 186:38 | attention to these differences for a long time until. And basically, |
|
| 186:46 | reason is because we don't speak but starting in 05, this was |
|
| 186:51 | clear to all of us um from um 2005 because we all speak |
|
| 187:00 | Um English is the universal language of and business all over the world. |
|
| 187:07 | know, because of the outcome of World War Two, if the Germans |
|
| 187:12 | won World War two right now, would all be speaking German and we |
|
| 187:17 | have seen this difference immediately in 1951 we didn't see it because we spoke |
|
| 187:25 | . So there's a, there's a here. This reveals a logical error |
|
| 187:29 | by gas mot and that logical error only uh um uh pointed out very |
|
| 187:37 | . So where did he go Well, here's where he went wrong |
|
| 187:41 | his derivation. He applied a theorem love that this is the same guy |
|
| 187:46 | who invented love waves. I told he was the last physicist who took |
|
| 187:53 | seriously. And for the whole book 1927 you can, it's since been |
|
| 187:59 | . You can, you can get money and you can get Love's book |
|
| 188:02 | on it on um on Amazon right . And so in there, love |
|
| 188:09 | a theorem which uh assumes a hydraulically system with food taken out of the |
|
| 188:16 | . He assumed he applied that theorem wave propagation which doesn't have the external |
|
| 188:23 | . The theorem is valid exactly valid case the ho the solid is, |
|
| 188:29 | micro homogeneous and approximately valid for the heterogeneous solid like like you know, |
|
| 188:36 | many, many minerals like a but it can't be applied to the |
|
| 188:41 | system of wave propagation since it assumes open system and is only valid in |
|
| 188:47 | context. That is Love's theorem. Gasman made a mistake when he applied |
|
| 188:52 | theorem to wave propagation. Yeah, might be thinking, well, Bo |
|
| 189:02 | the same mistake because he's got in the, the, the in the |
|
| 189:07 | of his frame it. And so true, we can measure the compressibility |
|
| 189:13 | the frame by measuring the co compressibility the drain system of the drained |
|
| 189:19 | So we need to uh ask ourselves question is the derivation also invalid because |
|
| 189:27 | showing in there the, the, frame that um and the compressibility of |
|
| 189:33 | frame which can be measured by allowing water to drain out. So, |
|
| 189:41 | there a problem here? Well, uh this problem is actually solved by |
|
| 189:48 | more guys, Brown and Kinga in again a long time ago. And |
|
| 189:54 | to show you how we're getting into modern era. Uh I know these |
|
| 189:58 | guys, I, I met they were working for Chevron when I |
|
| 190:03 | , uh, uh, working as post doc in Pasadena at Caltech. |
|
| 190:10 | , uh, uh, me and , and a buddy went to, |
|
| 190:14 | , uh, Chevron at the research , uh, about 50 miles |
|
| 190:18 | We went there and we talked to guys. Brown was, um, |
|
| 190:22 | employer of Chevron and Kinga was a at Ohio State, a professor of |
|
| 190:28 | at Ohio State who used to spend summers at Chevron consulting with. And |
|
| 190:35 | they did this work in 1975 and they uh considered more complex situation than |
|
| 190:45 | . Uh gasoline did uh they uh also the case of rocks with uh |
|
| 190:51 | and minerals so that the solid is Mike is heterogeneous on the microscopic |
|
| 191:00 | . And so here is uh uh their paper and here is the result |
|
| 191:07 | they result that this is uh without the logical error that gas mine |
|
| 191:12 | they did not use that formula from uh theorem from love. And so |
|
| 191:20 | you look at this, you see bunch of strange grammars, you see |
|
| 191:25 | that you don't recognize. Kappa What does that mean? Kappa |
|
| 191:29 | What does that mean? Kappa What does that mean? Well, |
|
| 191:33 | Kappa F, we sort of recognize that's the compressibility of the fluid. |
|
| 191:38 | Kappa Phi, what is that? , all these strange paras uh uh |
|
| 191:44 | uh shame on these guys. They smart guys, but they lose notation |
|
| 191:49 | confused people for years and years. , this Brown and Kinga formula in |
|
| 191:58 | is exactly equivalent to Bo and they cite bo A uh actually, it |
|
| 192:04 | has a different notation. So let's uh so this is bo uh uh |
|
| 192:08 | this has notation uh uh which is well, let's see here. If |
|
| 192:17 | look at the comparison here, you see that Kappa star obviously means the |
|
| 192:22 | Kappa. Kappa A obviously means the of the compressibility of the frame. |
|
| 192:29 | didn't they just call it frame? But then uh uh these two here |
|
| 192:35 | to the other two. The other notations in BRA and ka. Uh |
|
| 192:44 | uh H inverse is obviously uh this here. And down here, you |
|
| 192:51 | uh uh uh Kappa Phi is obviously to uh to uh our inverse. |
|
| 192:58 | I have it wrong. You have multiply it by a minus one and |
|
| 193:02 | a Phi and then we get our . But the point here is that |
|
| 193:07 | have the same number of verbs, number of verb gas mo reduced the |
|
| 193:16 | of verbals by one that was a error by gas. But, and |
|
| 193:24 | uh at least uh um now we agreement by these two groups uh uh |
|
| 193:31 | independently and let's find, figure out these other, no other uh uh |
|
| 193:38 | mean. So uh first, let's uh the asymptotic limiting case where we're |
|
| 193:47 | at the undrained in compressibility, which mean the rein test test as a |
|
| 193:54 | of the compressibility of the fluid when goes to infinity. So that means |
|
| 193:59 | uh changing um brine to gas when the, the gas has infinite |
|
| 194:09 | , it has zero in compressibility and has infinite compressibility. And so, |
|
| 194:15 | this term goes to infinity, the thing uh uh goes to zero. |
|
| 194:21 | then we uh uh we realize that A refers to the asymptotic limiting case |
|
| 194:30 | um the of the, the compressibility the fluid is infinite. So we're |
|
| 194:39 | interpret this A. So in that , the load is supported, owned |
|
| 194:44 | the Frank. Therefore, uh Kappa equals Kappa Frank. So it |
|
| 194:51 | although it can be measured as the compressibility, it's defined by the functional |
|
| 194:58 | of the undrained rock upon the f fluid and compressibility. It's the asymptotic |
|
| 195:06 | this expression. So that's Kappa What, what is Kappa N? |
|
| 195:15 | very tempting to think of Kappa N oh, that's the uh the, |
|
| 195:19 | compressibility of the meals. No, brown and Kinga provide this expression with |
|
| 195:29 | in their form in their paper, uh they provide this expression which, |
|
| 195:34 | uh connects Kappa M with the average of the solid. That's the |
|
| 195:42 | that's the average. And here is compressibility of the pore space. So |
|
| 195:50 | words, cap M is the volume average of the solid in the pore |
|
| 195:56 | . So we're gonna interpret that M from, that's the mean compressibility, |
|
| 196:01 | the mine compress reality. Mine mental compression compressibility is given by Kas |
|
| 196:09 | for solid. So now we can these two expressions by gas mo and |
|
| 196:14 | Brown and Kinga. And we can that uh uh uh this comparison here |
|
| 196:20 | uh here uh uh gas mo has the logical error made by gas mot |
|
| 196:29 | in the replacement of Kappa M by S here and also over here. |
|
| 196:36 | uh and it uh uh results in replacement of Kappa Phi by Kappa S |
|
| 196:43 | . So that's how he reduced the of variables from uh uh uh from |
|
| 196:49 | to 2 by assuming that these three , these three quantities are all the |
|
| 196:58 | . So no uh right here in , in the text that I just |
|
| 197:04 | here. And it says Ron and argues that if the solid is micro |
|
| 197:10 | , then these three are all the . In that case, their result |
|
| 197:15 | to gas mot. So because they that argument, everybody has assumed ever |
|
| 197:22 | 1975. Ever since this came out 1975 they assumed that these differences, |
|
| 197:28 | are now clear to everybody, those are due to the micro heterogeneity which |
|
| 197:34 | and Kinga uh introduced. And so argued that if the solid is not |
|
| 197:40 | heterogeneous, but if it's micro homo only one isotropic mineral. Then they |
|
| 197:49 | that these three are the same. so their result reduces to the uh |
|
| 197:57 | uh to the uh gas line However, this argument that they made |
|
| 198:06 | argument there, they made the same as Gasman did. They applied Love's |
|
| 198:12 | . But her Sims um um open , they applied that to the bra |
|
| 198:19 | so that this argument, this particular is uh uh is wrong. So |
|
| 198:25 | got to use Brown Fringer result above one here in all cases and homogeneous |
|
| 198:33 | and homogeneous or not. So that a complication for us in applying these |
|
| 198:43 | to four sizes. Normally, when uh uh make uh an interpretation of |
|
| 198:54 | four D differences that we see in four D seismic data, we |
|
| 199:01 | that, that the compressibility of the might be changing as we're changing Brian |
|
| 199:06 | oil and gas or vice or whatever doing. Uh But this one is |
|
| 199:10 | changing and we assume that we know the solid is and how do we |
|
| 199:15 | what the solid is? Um uh , we don't really know what the |
|
| 199:19 | is, we, we say. . Uh We think we know what |
|
| 199:24 | solid is and we can look up properties of that solid in a |
|
| 199:30 | How do we do that? we think we know what the solid |
|
| 199:34 | , we know what the mineral composition . And so we know uh so |
|
| 199:39 | corp, so much legislation and so . Look up in uh a |
|
| 199:43 | what are the properties of those minerals uh uh do a voice calculation and |
|
| 199:49 | a voice calculation and do a hill of those uh two things. And |
|
| 199:54 | gonna give us the solid uh uh . I know that you are thinking |
|
| 200:00 | a lot of, of uh of possibilities for error built into that, |
|
| 200:08 | that's what we always do. And , we do the same thing uh |
|
| 200:13 | and we do the same thing We assume that the uh the compressibility |
|
| 200:18 | the pore space is the same as compressibility of the, the mean compressibility |
|
| 200:24 | the same as the compressibility of the . Now, I know what you're |
|
| 200:31 | . You're thinking it's not possible for pore space to have the same compressibility |
|
| 200:36 | the solid solid is, you solid grains, the pore space is |
|
| 200:42 | uh is not or space. Let's it's got a fluid in there. |
|
| 200:48 | say it's got AAA brine in Uh But the bo space is gonna |
|
| 200:54 | formed separately because um the Brian uh different compressibility than the solid. |
|
| 201:11 | And you could be right. Uh me carry on the argument and let's |
|
| 201:20 | how we're gonna uh determine these How are we gonna determine the main |
|
| 201:31 | and the poor compressibility? It's well by our friends who do laboratory experiments |
|
| 201:38 | this is a hard quantity to But here's the good news is we |
|
| 201:44 | need to do, we don't need measure it because we have the previous |
|
| 201:48 | from uh Brown and Kinga relating the compressibility to the mean compressibility and the |
|
| 201:55 | compressibility. So when we um reckon we take that expression and put it |
|
| 202:03 | brown and Kroger's result, we, see no nowhere do, do we |
|
| 202:08 | the poor compressibility because we substituted this . And now we see only the |
|
| 202:14 | compressibility and the sot compressibility. That's we have to uh determine. So |
|
| 202:20 | first question is how, how do determine the mean compressor? Bet? |
|
| 202:24 | , that's easy. Also go back bo and the, the first impression |
|
| 202:29 | I showed from bio uh showed that um um uh B OS parameter H |
|
| 202:40 | given in terms of the frame compressibility the mean compressibility this way. So |
|
| 202:45 | just solve this expression for the uh the mean compressibility. And this is |
|
| 202:51 | we find in terms of things which observable and on my contract explicitly on |
|
| 203:04 | um ratio here of the fluid pressure the external pressure undrained. But that's |
|
| 203:11 | you can measure. Take a porous , fill it with bri we put |
|
| 203:17 | , a membrane around the outside. nothing that squeezes in or out, |
|
| 203:22 | uniform pressure on all sides and then yourself, what is the pore pressure |
|
| 203:29 | the inside? Well, you can measure that you make a little hole |
|
| 203:33 | the membrane and put a AAA little through that hole and measure the pressure |
|
| 203:40 | uh in the, in the fluid , make sure the pipe ends up |
|
| 203:45 | a pore space. And so you measure the pore fluid in that |
|
| 203:50 | Uh It's not easy, but it be done. Uh People have been |
|
| 203:53 | that for years and then that gives then the mean compressibility. I, |
|
| 204:07 | , I've, I've, I've not uh shown you here how to uh |
|
| 204:14 | how to find how to find the compressibility in a porous rock. What |
|
| 204:22 | don't wanna do is uh grind up finely and squeeze that because no matter |
|
| 204:27 | f how uh how fine you grind , there's always gonna be uh inter |
|
| 204:35 | cracks there. And furthermore, you destroyed the uh original micro geometry of |
|
| 204:42 | uh of the rock. So that's not what you wanna do. |
|
| 204:47 | There, there is a way to the solid. Um There is a |
|
| 204:54 | to measure the solid compressibility of the without destroying the rock. That's a |
|
| 205:00 | and like physics. So uh uh , sorry, I have a |
|
| 205:09 | Maybe if we are very ahead, I should have asked before. But |
|
| 205:15 | , what's the difference between the, frame and the solid here in the |
|
| 205:21 | compressibility? Because I understand that the the frame is when it's drained, |
|
| 205:29 | ? But the solid, it, is also the minerals, but it's |
|
| 205:34 | the brand, right. Well, when you measure that fra that drain |
|
| 205:40 | , uh you know, you're uh the pore space as well as the |
|
| 205:45 | . And so you're not measuring the of the solid, you're measuring the |
|
| 205:51 | of the, of, of the . So to measure the compressibility of |
|
| 205:58 | solid itself without considering any of the um uh of the course, uh |
|
| 206:07 | , I told you that I'm not to uh explain how to do |
|
| 206:13 | Uh uh rock physicists know how to that. But since this is the |
|
| 206:18 | and wave propagation, I'm, I'm gonna uh to tell you that. |
|
| 206:24 | here's, I if you're interested in topic and I'm happy that you are |
|
| 206:29 | uh I can refer you to a contribution, recent paper that I |
|
| 206:36 | And I'm the one who uh who that Gasman made this logical error. |
|
| 206:44 | I published a paper last year uh explaining all this. And I, |
|
| 206:51 | , if you're interested now I'll send a copy, uh just uh write |
|
| 206:56 | me or you can ask me send you a copy and I can |
|
| 207:00 | you that it was uh uh not to get this paper published. I'll |
|
| 207:05 | tell you uh uh uh uh we a few minutes before the break. |
|
| 207:09 | , I'll tell you what happened. I thought about this for many |
|
| 207:13 | And as I thought about it, uh the uh the issues became more |
|
| 207:18 | more clear in my mind. And finally um uh was ready for |
|
| 207:24 | So I about in the year 20 I submitted it for publication. And |
|
| 207:33 | of course, I submitted it to which is the leading journal in our |
|
| 207:38 | published by the SCG. So it rejected by the uh reviewers. And |
|
| 207:46 | uh um uh you, you might that um especially in for the journal |
|
| 207:54 | uh when you submit a paper to uh um to the journal, what |
|
| 208:00 | editor does was is he uh uh make a judgment about this himself. |
|
| 208:06 | he has lots of uh of uh who help him and he has associate |
|
| 208:11 | and he's got reviewers and so on the reviewers are experts in the subject |
|
| 208:17 | , but they are selected by the and by the associate editor so that |
|
| 208:22 | uh have no bias that is they not um working for the same company |
|
| 208:29 | the um as the author. I it's a small community of rock |
|
| 208:34 | and we all know each other. they're bou we're bound to be |
|
| 208:38 | But uh uh uh uh in, order to avoid uh the uh uh |
|
| 208:49 | issues that could be raised by this , what the editor does, the |
|
| 208:54 | of Geophysics, he takes the author's off of the paper and he sends |
|
| 208:59 | to the reviewers and the reviewer uh doesn't know who wrote the paper. |
|
| 209:06 | might be able to figure it But uh uh it, it |
|
| 209:09 | he, he's always uh not quite who published this paper. He looks |
|
| 209:13 | this paper that I submitted. There many of them and they all said |
|
| 209:18 | is nonsense. Everybody knows that Gas is correct. We've all been using |
|
| 209:23 | Mon for uh 70 years in uh paper is wrong. We uh recommend |
|
| 209:29 | the editor reject it. And after arguments with the reviewers and with the |
|
| 209:36 | and with the associate editors, all whom were friends of mine, they |
|
| 209:40 | the Baker B uh oh Leon, just wrong here. So I didn't |
|
| 209:49 | up. Uh I started presenting the at um uh at the convention, |
|
| 209:56 | convention of the SCG and the convention the uh uh Eage, which is |
|
| 210:03 | second most important society that we And I also submitted it for publication |
|
| 210:10 | uh by the Eage. You they have their own editors and their |
|
| 210:15 | referees and, and, and they rejected it also similar start. |
|
| 210:20 | knows that Gas Mi is great. so uh meanwhile, I'm, I'm |
|
| 210:26 | resenting it and I'm presenting it. And I'm, I'm, I'm winning |
|
| 210:33 | in, in 1921. I think won an award for the best presentation |
|
| 210:38 | this idea at the seg. So expected that uh editor would look at |
|
| 210:48 | . And uh uh and he would , oh, that paper which I |
|
| 210:53 | last year has been uh recognized by the society as the best paper presented |
|
| 211:00 | in the entire seg convention in But he didn't do that. So |
|
| 211:09 | , I'm also presenting it at the at those uh reviewers are uh uh |
|
| 211:16 | it very highly. But the Eage saw those high reviews and he was |
|
| 211:23 | to get a good paper into his . And he said you should submit |
|
| 211:28 | to the journal again. And I , well, you, you rejected |
|
| 211:32 | uh once already. And he uh uh uh uh never mind uh |
|
| 211:38 | uh answer all the objections of the gave and submit it again. So |
|
| 211:45 | what I did again. And so uh again, the, the referees |
|
| 211:51 | objections, but I answered them And after a lot of back and |
|
| 211:56 | , it was finally accepted for publication the Eage and their flagship journal, |
|
| 212:02 | is called Geophysical Prospecting of last And so if you're interested, you've |
|
| 212:08 | uh uh got a copy of that directly from the journal or for |
|
| 212:16 | be happy to do it. And so this is all now out |
|
| 212:20 | in the open. And you can that there's a lot of controversy, |
|
| 212:26 | lot of people saying what gas mon wrong. How could that possibly be |
|
| 212:32 | ? Uh And then uh uh um what are we gonna do? Suppose |
|
| 212:40 | take this seriously and, and, uh uh that you use this |
|
| 212:47 | which is basically the expression given by and Kinga. But it's got in |
|
| 212:52 | the mean compressibility. How are we to know what to put in there |
|
| 212:58 | our reservoir? 10,000 ft now? , basically it's the same problem as |
|
| 213:03 | have here. Uh with the solid you have to have a laboratory experiments |
|
| 213:11 | assume that, that the uh uh that the, the rock you have |
|
| 213:16 | the laboratory is the same as you down there in the reservoir for determining |
|
| 213:24 | the solid compressibility and the uh green . It's obviously open to uh uh |
|
| 213:34 | . Somebody can always say, oh a different rock. So what we |
|
| 213:38 | to do is we have to do sorts of experiments on lots, lots |
|
| 213:42 | rocks. So we get to know are uh what uh what are |
|
| 213:47 | the properties of uh lots of rocks lots of pressure conditions, lots of |
|
| 213:53 | conditions, lots of ages and so and have a large database of these |
|
| 213:59 | of measurements to um draw from when trying to analyze four D data. |
|
| 214:07 | a big experimental program. And I uh uh I believe that rock properties |
|
| 214:17 | all over the world are taking up challenge. To measure both Kappa M |
|
| 214:25 | Kappa S in the laboratory for lots rocks. And I'm thinking that in |
|
| 214:30 | or 10 years, we'll have enough to design whether um uh uh what |
|
| 214:39 | of numbers we should be using in subsurface. So let me um uh |
|
| 214:45 | forward here. Uh All of this about uh compressibility. So it's easy |
|
| 214:53 | uh to change. Um uh uh result which I just showed uh into |
|
| 215:02 | compressibility, that's what we need for wave propagation. And the formula looks |
|
| 215:07 | much like Gas Mon's formula. But of having the inverse of, |
|
| 215:12 | of KPs here, we have Kappa and we have Kappa M down |
|
| 215:19 | And so we're challenged with determining both those. And you can see that |
|
| 215:24 | Kappa M reduces to Kappa S, is the same as the inverse of |
|
| 215:30 | S, then this reduces to And so the whole question resolves is |
|
| 215:36 | similar is Kappa M to Kappa What is this? How, how |
|
| 215:43 | is this difference? Well, it upon lots of experiments not yet |
|
| 215:54 | So, oh You will find the discussion this last uh perhaps an hour |
|
| 216:02 | discussion about the effects of fluids on velocities to be um uh sort of |
|
| 216:11 | because it's introducing comp uh complication, it's not resolving uh those issues. |
|
| 216:22 | , I'll remind you what we said that uh ordinary waves are ordinary or |
|
| 216:29 | waves propagate through rocks just like we talking about earlier according to the previous |
|
| 216:35 | . But what we now know is this uh uh the in compressibility parameter |
|
| 216:41 | upon on the I in compressibility of K. And the same thing for |
|
| 216:46 | density but not for the sheer So the good news is that we |
|
| 216:54 | just use all of the elastic uh using the poor elastic constant k, |
|
| 217:01 | and density undrained. And furthermore, know that there's more complexity due to |
|
| 217:12 | uh fluids pose the fluid is not , suppose that we have a mixture |
|
| 217:17 | fluids. Then we also know from work that we can actually use the |
|
| 217:23 | formula for in compressibility. That's what showed before and that's exact for |
|
| 217:34 | Now, uh what are these different compressibility for uh for brine? |
|
| 217:43 | the incompressible for brine depends in a way on the salinity of the |
|
| 217:49 | We can ignore that it depends in significant way on the composition of the |
|
| 217:54 | . Like how much light fraction of , how much heavy fraction of oil |
|
| 217:57 | have here. But the main issue this comes from the in the compressibility |
|
| 218:02 | the gas. And it's known that , if there is an as much |
|
| 218:07 | 1% of the gas. So if is is as large as 1% then |
|
| 218:14 | the compression, the incompressible of gas so small, we got a small |
|
| 218:19 | here in the denominator. And that this term dominates the east term. |
|
| 218:27 | that's affecting uh uh uh the compressibility the fluid in a major way that |
|
| 218:33 | the velocity in a major way because compressibility is coming in right here. |
|
| 218:39 | then we know from previous slide that velocity has this in compressibility, that's |
|
| 218:47 | uh uh that's this one. And depends upon the fluid compressibility and the |
|
| 218:53 | that's shown here. And because of chain of logic, seismic data is |
|
| 219:00 | a good quantitative predictor of gas Why is that? Because un uneconomic |
|
| 219:10 | of gas, like 1% of gas appear seismically to be the same as |
|
| 219:16 | of gas. So it can uh it can appear seismically to be economic |
|
| 219:26 | of gas even though it's maybe 1% gas. And the rest is |
|
| 219:31 | you don't wanna drill into that. there have been many cases where we |
|
| 219:36 | used a bo to predict the presence uh hydrocarbon in the subsurface. And |
|
| 219:44 | we drill there, we find that just a little bit of gas in |
|
| 219:55 | . Summarizing all that discussion, the velocity of Iran decreases significantly with saturated |
|
| 220:05 | . And when we talk here, know, let's let's um back up |
|
| 220:13 | significantly with saturated gas because if it's with gas, uh uh then uh |
|
| 220:20 | only contribution to the uh compressibility, in compressibility of the rock comes from |
|
| 220:27 | frame. Whereas if there's Brian in a much bigger number, this is |
|
| 220:35 | under uh uh uh uh the rein compressibility of the uh dry and saturated |
|
| 220:45 | . That's a greater number. So of, because of this ratio of |
|
| 220:49 | uh inequality right here, that's a number than this one. What uh |
|
| 220:57 | these other uh issues uh of uh uh oh And here's the new issue |
|
| 221:03 | I didn't say I have, I said that the, the in compressibility |
|
| 221:09 | gas is very small, but maybe not true. Maybe under poor pressure |
|
| 221:16 | at the under reservoir conditions, maybe , the pressure in a reservoir is |
|
| 221:21 | high that the compressibility of the gas there is uh not so negligible natural |
|
| 221:32 | . So uh um that's why we to have um uh serious treatments of |
|
| 221:46 | properties of fluids under high pressure and temperature to understand what fluid number to |
|
| 221:56 | into. I'm gonna back up What fluid number should go into |
|
| 222:03 | If we, if we have AAA reservoir with a complex chemistry of uh |
|
| 222:10 | in there, you need to ask what is the uh uh com the |
|
| 222:16 | of those fluids? So you'll know number to put in here. Oh |
|
| 222:22 | the same number that's going in Um uh So we have, we |
|
| 222:28 | know the answer to that question depends the composition and uh uh uh rock |
|
| 222:35 | laboratories like we have here at the of Houston, know what that relation |
|
| 222:40 | . Uh It's a result of a of work by a lot of people |
|
| 222:43 | many years. And so you have uh uh talk to those people before |
|
| 222:48 | decide what is the composition of the portion down there? Yeah. |
|
| 222:59 | Density. Oh yeah. So I think the density is pretty |
|
| 223:03 | So uh uh the density is We talked about that before. Uh |
|
| 223:08 | the in compressibility which is hard. so that's why we're talking about |
|
| 223:12 | So this leads to many effects, uh this is a good time for |
|
| 223:16 | to break right now. So I'm to leave this at this point and |
|
| 223:20 | come back to you the effects of um uh uh inequality right here, |
|
| 223:28 | back after lunch. So let's break lunch at um we'll come back at |
|
| 223:33 | o'clock, Houston time. And so am going to s uh I sign |
|
| 223:38 | , let's see here. The way gonna do it is uh um um |
|
| 223:47 | am going to uh and the slideshow then I'm gonna stop sharing. And |
|
| 223:57 | uh I think we'll leave uh uh um uh you should leave, stop |
|
| 224:03 | recording |
|